No CrossRef data available.
Published online by Cambridge University Press: 18 January 2015
Let K0(Vark) denote the Grothendieck ring of k-varieties over an algebraically closed field k. Larsen and Lunts asked if two k-varieties having the same class in K0(Vark) are piecewise isomorphic. Gromov asked if a birational self-map of a k-variety can be extended to a piecewise automorphism. We show that these two questions are equivalent over any algebraically closed field. If these two questions admit a positive answer, then we prove that its underlying abelian group is a free abelian group and that the associated graded ring of the Grothendieck ring is the monoid ring 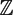 $\mathbb{Z}$[
$\mathbb{Z}$[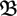 $\mathfrak{B}$] where
$\mathfrak{B}$] where 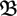 $\mathfrak{B}$ denotes the multiplicative monoid of birational equivalence classes of irreducible k-varieties.
$\mathfrak{B}$ denotes the multiplicative monoid of birational equivalence classes of irreducible k-varieties.