Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Munn, W. D.
1957.
Matrix representations of semigroups.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 53,
Issue. 1,
p.
5.
Munn, W. D.
1957.
Semigroups satisfying minimal conditions.
Proceedings of the Glasgow Mathematical Association,
Vol. 3,
Issue. 3,
p.
145.
Munn, W. D.
1961.
A Class of Irreducible matrix representations of an Arbitrary Inverse Semigroup.
Proceedings of the Glasgow Mathematical Association,
Vol. 5,
Issue. 1,
p.
41.
Lallement, Gérard
and
Petrich, Mario
1969.
Irreducible matrix representations of finite semigroups.
Transactions of the American Mathematical Society,
Vol. 139,
Issue. 0,
p.
393.
Weissglass, Julian
1969.
Radicals of semigroup rings.
Glasgow Mathematical Journal,
Vol. 10,
Issue. 2,
p.
85.
Ponizovskii, I. S.
1971.
On quasi-Frobeniusity of semigroup algebras of finite regular semigroups.
Siberian Mathematical Journal,
Vol. 11,
Issue. 6,
p.
967.
McAlister, D. B.
1971.
The category of representations of a completely 0-simple semigroup.
Journal of the Australian Mathematical Society,
Vol. 12,
Issue. 2,
p.
193.
McAlister, D. B.
1971.
Rings related to completely 0-simple semigroups.
Journal of the Australian Mathematical Society,
Vol. 12,
Issue. 3,
p.
257.
Janeski, John
and
Weissglass, Julian
1973.
Regularity of semilattice sums of rings.
Proceedings of the American Mathematical Society,
Vol. 39,
Issue. 3,
p.
479.
Weissglass, Julian
1973.
Semigroup rings and semilattice sums of rings.
Proceedings of the American Mathematical Society,
Vol. 39,
Issue. 3,
p.
471.
Zalesskii, A. E.
and
Mikhalev, A. V.
1975.
Group rings.
Journal of Soviet Mathematics,
Vol. 4,
Issue. 1,
p.
1.
Barnes, Bruce A.
1976.
Representations of the 𝑙¹-algebra of an inverse semigroup.
Transactions of the American Mathematical Society,
Vol. 218,
Issue. 0,
p.
361.
Munn, W.D.
1980.
Semigroups.
p.
207.
Márki, L.
and
Wiegandt, R.
1981.
Semigroups.
Vol. 855,
Issue. ,
p.
55.
Munn, W. D.
1982.
Semiprimitivity of inverse semigroup algebras.
Proceedings of the Royal Society of Edinburgh: Section A Mathematics,
Vol. 93,
Issue. 1-2,
p.
83.
Okniński, Jan
1984.
Semilocal semigroup rings.
Glasgow Mathematical Journal,
Vol. 25,
Issue. 1,
p.
37.
Ching-an Cheng, Charles
1984.
Separable semigroup algebras.
Journal of Pure and Applied Algebra,
Vol. 33,
Issue. 2,
p.
151.
Munn, W.D.
1986.
Group and Semigroup Rings, Centro de Brasileiro de Pesquisas Fisicas Rio de Janeiro and University of Rochester.
Vol. 126,
Issue. ,
p.
197.
Ponizovskiî, J. S.
1987.
Semigroup rings.
Semigroup Forum,
Vol. 36,
Issue. 1,
p.
1.
Munn, W. D.
1987.
Semigroups and Their Applications.
p.
111.


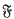 , the concept of the group algebra (group ring) over
, the concept of the group algebra (group ring) over 