Hostname: page-component-78c5997874-mlc7c Total loading time: 0 Render date: 2024-11-08T05:05:49.610Z Has data issue: false hasContentIssue false
  • English
  • Français

On elements of order four in certain free central extensions of groups

Published online by Cambridge University Press:  24 October 2008

Ralph Stöhr
Ralph Stöhr
Affiliation:
Akademie der Wissenschaften der DDR, Karl- Weierstraβ-Institut für Mathematik, Mohrenstraβe 39, Berlin, DDR-1086, German Democratic Republic
Get access Rights & Permissions [Opens in a new window]

Extract

Let F be a non-cyclic free group, R a normal subgroup of F and G = F/R, i.e.

where π is the natural projection of F onto G, is a free presentation of G. Let R′ denote the commutator subgroup of R. The quotient F/[R′,F] is a free central extension

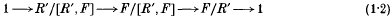
of the group F/R′, the latter being a free abelianized extension of G. While F/R′ is torsion-free (see, e.g. [2], p. 23), elements of finite order may occur in R′/[R′,F], the kernel of the free central extension (l·2). Since C. K. Gupta [1] discovered elements of order 2 in the free centre-by-metabelian group F/[F″,F] (i.e. (1·2) in the case R = F′), torsion in F/[R′,F] has been studied by a number of authors (see, e.g. [4–13]). Clearly the elements of finite order in F/[R′,F] form a subgroup T of the abelian group R′/[R′,F]. It will be convenient to write T additively. By a result of Kuz'min [5], any element of T has order 2 or 4. Moreover, it was pointed out in [5] that elements of order 4 may really occur. On the other hand, it has been shown in [11] that, if G has no 2-torsion, then T is an elementary abelian 2-group isomorphic to H4(G, ℤ2). So if T contains an element of order 4, then G must have 2-torsion. We also mention a result of Zerck [13], who proved that 2T is an invariant of G, i.e. it does not depend on the particular choice of the free presentation (1·1).

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 106 , Issue 1 , July 1989 , pp. 13 - 28
Copyright
Copyright © Cambridge Philosophical Society 1989

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Gupta, C. K.. The free centre-by-metabelian groups. J. Austral. Math. Soc. 16 (1973), 294–300.CrossRefGoogle Scholar
[2]Gupta, N. D.. Free Group Rings. Contemp. Math. vol. 66 (American Mathematical Society, 1987).CrossRefGoogle Scholar
[3]Hilton, P. and Stammbach, U.. A Course in Homological Algebra (Springer-Verlag, 1971).CrossRefGoogle Scholar
[4]Kuz'min, Yu. V.. Free center-by-metabelian groups, Lie algebras and D-groups. Izv. Akad. Nauk SSSR Ser. Mat. 41 (1977), 3–33.Google Scholar
[5]Kuz'min, Yu. V.. On elements of finite order in free groups of some varieties. Mat. Sb. (N.S.) 119 (1982), 119–131.Google Scholar
[6]Kuz'min, Yu. V.. The structure of the free groups of some varieties. Mat. Sb. (N.S.) 125 (1984), 128–142.Google Scholar
[7]Kuz'min, Yu. V.. Elements of order 2 in the free center-by-soluble group. Mat. Zametki 37 (1985), 643–652.Google Scholar
[8]Kuz'min, Yu. V.. Homology theory of free abelianized extensions. Comm. Algebra 16 (1988), 2447–2533.CrossRefGoogle Scholar
[9]Lewin, J. and Lewin, T.. On center by abelian by one-relator groups. Comm. Pure Appl. Math. 26 (1973), 767–774.CrossRefGoogle Scholar
[10]Stöhr, R.. On Gupta representations of central extensions. Math. Z. 187 (1984), 259–267.CrossRefGoogle Scholar
[11]Stöhr, R.. On torsion in free central extensions of some torsion-free groups. J. Pure Appl. Algebra 46 (1987), 249–289.Google Scholar
[12]Thomson, M. W.. Representations of certain verbal wreath products by matrices. Math. Z. 167 (1979), 239–257.Google Scholar
[13]Zerck, R.. On the homology of the higher relation modules. J. Pure Appl. Algebra (to appear).Google Scholar