Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-25T02:35:36.752Z Has data issue: false hasContentIssue false
  • English
  • Français

On a Class of Tchebysheffian Approximation Problems Solvable Algebraically

Published online by Cambridge University Press:  24 October 2008

A. Talbot
A. Talbot
Affiliation:
Imperial CollegeLondon
Get access Rights & Permissions [Opens in a new window]

Extract

The general Tchebysheffian approximation problem is the following: Given a real continuous function g(x) in the interval a ≤ x ≤ b, and a real function f(x; Pj) of prescribed form, continuous in the variable x and the parameters Pj, determine the values of the parameters so that the measure of absolute error or ‘deviation’ of f, 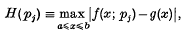shall be as small as possible.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 58 , Issue 2 , April 1962 , pp. 244 - 267
Copyright
Copyright © Cambridge Philosophical Society 1962

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Tchebysheff, P. L.Sur les questions de minima qui se rattachent á la représentation approchée des fonotions. Mem. Acad. Imp. Sci. St-Pétersburg, IX (1859), 201–291; Oevres, I, 273–378.Google Scholar
(2)Achieser, N. ITheory of approximation (New York, 1956), Chapter II.Google Scholar
(3)Abel, N. HRemarques sur quelques propriétés générales des fonctions transcendantes. J. Reine Angew. Math. 3 (1828); Oevres, I, 444–456.Google Scholar
(4)Abel, N. HSur l'intégration de la formule différentielle pdx / √ R.. J. Reine Angew. Math. 1 (1826), 185–221; Oevres, I, 104–144.Google Scholar
(5)Bernstein, S.Lecons sur les propriétés exirémales et la meilleure approximation des fonctions analytiques d'une variable réelle (Paris, 1926).Google Scholar
(6)Tchebysheff, P. LSur les polynomes représentant les mieux les valeurs des fonctions fractionnaires élémentaires. Mem. Acad. Imp. Sci. St-Pétersburg, 72 (1893) (Suppl.), no. 7; Oevres II, 669.Google Scholar
(7)Achieser, N. IUber ein Tchebysheffsches Extremumproblem. Math. Ann. 104 (1931), 739.CrossRefGoogle Scholar