Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-25T00:50:57.433Z Has data issue: false hasContentIssue false
  • English
  • Français

A note on the strong regularity of Nrlund means

Published online by Cambridge University Press:  24 October 2008

J. R. Nurcombe
J. R. Nurcombe
Affiliation:
Hall Green Technical College, Colebank Road, Birmingham 28
Get access Rights & Permissions [Opens in a new window]

Extract

Let (pn), (qn) and (un) be sequences of real or complex numbers with

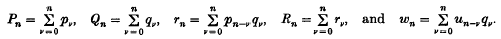
The sequence (sn) is strongly generalized Nrlund summable with index 0, to s, or s or snsN, p, Q if

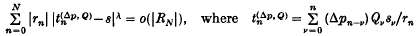
and pnv=pnvpnv1, with p10. Strong Nrlund summability N, p was first studied by Borweing and Cass (1), and its generalization N, p, Q by Thorp (6). We shall say that (sn) is strongly generalized convergent of index 0, to s, and write snsC, 0, Q if sns and 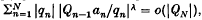where sn=a0+a1++an. When qn all n, this definition reduces to strong convergence of index , introduced by Hyslop (4). If as n, the sequence (sn) is summable (, q) to s sns(, q).

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 94 , Issue 2 , September 1983 , pp. 261 - 263
Copyright
Copyright © Cambridge Philosophical Society 1983

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Borwein, D. and Cass, F. P.Strong Nrlund summability. Math. Z. 103 (1968), 94111.CrossRefGoogle Scholar
(2)Das, G. On some methods of summability (II). Quart. J. Math. Oxford Ser 19 (1968), 417431.CrossRefGoogle Scholar
(3)Hardy, G. HDivergent series (Oxford, 1949).Google Scholar
(4)Hyslop, J. M.Note on the strong summability of series. Proc. Glasgow Math. Assoc. 1 (1952), 1620.CrossRefGoogle Scholar
(5)Kuttner, B.Some theorems on the strong regularity of Nrlund methods. Proc. Cambridge Philos. Soc. 76 (1974), 195209.CrossRefGoogle Scholar
(6)Doctoral, Thorpe B. thesis (University of Birmingham, 1969).Google Scholar