Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-24T12:12:49.734Z Has data issue: false hasContentIssue false
  • English
  • Français

A note on functions regular and bounded in the unit circle and small at a set of points near the circumference of the circle

Published online by Cambridge University Press:  24 October 2008

B. J. Maitland
B. J. Maitland
Affiliation:
Newnham CollegeCambridge
Get access Rights & Permissions [Opens in a new window]

Extract

The following theorem was proved by Milloux:

Theorem A. Suppose that f (z) is regular and that | f(z) | < 1 in the unit circle. Suppose also that the set of points in the circle | z | ≤ r′ < 1 at which

cannot be enclosed in circles, the sum of whose radii is equal to 2eh−1. Then

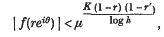
where K is a numerical constant.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 35 , Issue 3 , July 1939 , pp. 382 - 388
Copyright
Copyright © Cambridge Philosophical Society 1939

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

† Milloux, H., Mathematica, 4 (1930), 182–5.Google Scholar

† This theorem was suggested by a theorem of Hall, T. in his paper “Sur la mesure harmonique de certains ensembles”, Arkiv för Matematik, Astronomi och Fysik, 25 A, no. 28 (1937).Google Scholar Hall's theorem is concerned with functions regular and bounded in the half-plane R(z) > 0, and small at a set of points tending to the origin. This set may be as near as we please to the line R(z) = 0.