Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-24T02:12:01.684Z Has data issue: false hasContentIssue false
  • English
  • Français

The minimum of a non-homogeneous binary cubic form

Published online by Cambridge University Press:  24 October 2008

J. H. H. Chalk
J. H. H. Chalk
Affiliation:
Bedford CollegeLondon, N.W. 1
Get access Rights & Permissions [Opens in a new window]

Extract

1. Let f(x, y) = ax3 + bx2y + cxy2 + dy3 be a binary cubic form with real coefficients. According as the discriminant D = b2c2+ 18abcd − 4ac3 − 4db3 − 27a2d2 satisfies D ≥ 0 or D < 0, the form f(x, y) can be factorized into either three real, or one real and two conjugate complex, linear factors. A form f1(x, y) is said to be equivalent to f2(x, y) if there is an integral unimodular substitution on the variables x, y transforming f1(x, y) into f2(x, y). Thus f2(x, y) is equivalent to f1(x, y) and, in fact, the set of all forms with given discriminant can be divided into classes of equivalent forms. Davenport (3) has specified a member of each class which satisfies

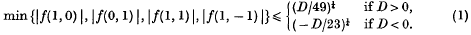
Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 48 , Issue 3 , July 1952 , pp. 392 - 401
Copyright
Copyright © Cambridge Philosophical Society 1952

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Bambah, R. P.Proc. Camb. phil. Soc. 47 (1951), 457–60.CrossRefGoogle Scholar
(2)Barnes, E. S.Quart. J. Math. (2), 1 (1950), 199–210.CrossRefGoogle Scholar
(3)Davenport, H.J. Lond. math. Soc. 20 (1945), 14–22.CrossRefGoogle Scholar
(4)Macbeath, A. M.Proc. Camb. phil. Soc. 47 (1951), 627–8.CrossRefGoogle Scholar
(5)Mordell, L. J.J. Lond. math. Soc. 17 (1942), 107–15.CrossRefGoogle Scholar
(6)Mordell, L. J.Proc. Lond. math. Soc. (2), 48 (1943), 198–228.Google Scholar
(7)Mordell, L. J.J. Lond. math. Soc. 19 (1944), 6–12.CrossRefGoogle Scholar
(8)Mordell, L. J.Proc. Lond. math. Soc. (2), 48 (1945), 339–90.CrossRefGoogle Scholar