Article contents
Meridian twisting of closed braids and the Homfly polynomial
Published online by Cambridge University Press: 01 May 2009
Abstract
Let β be a braid on n strands, with exponent sum w. Let Δ be the Garside half-twist braid. We prove that the coefficient of vw−n+1 in the Homfly polynomial of the closure of β agrees with (−1)n−1 times the coefficient of vw+n2−1 in the Homfly polynomial of the closure of βΔ2. This coincidence implies that the lower Morton–Franks–Williams estimate for the v–degree of the Homfly polynomial of 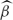 is sharp if and only if the upper MFW estimate is sharp for the v–degree of the Homfly polynomial of
is sharp if and only if the upper MFW estimate is sharp for the v–degree of the Homfly polynomial of 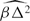 .
.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 146 , Issue 3 , May 2009 , pp. 649 - 660
- Copyright
- Copyright © Cambridge Philosophical Society 2008
References
REFERENCES
- 9
- Cited by


