Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-29T07:50:35.008Z Has data issue: false hasContentIssue false
  • English
  • Français

Johnson–Levine homomorphisms and the tree reduction of the LMO functor

Part of: Low-dimensional topology

Published online by Cambridge University Press:  27 November 2019

ANDERSON VERA
ANDERSON VERA*
Affiliation:
Institut de Recherche Mathématique Avancée Université de Strasbourg, 67084 Strasbourg cedex, France. e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Let $\mathcal{M}$ denote the mapping class group of Σ, a compact connected oriented surface with one boundary component. The action of 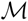$\mathcal{M}$ on the nilpotent quotients of π1(Σ) allows to define the so-called Johnson filtration and the Johnson homomorphisms. J. Levine introduced a new filtration of 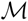$\mathcal{M}$, called the Lagrangian filtration. He also introduced a version of the Johnson homomorphisms for this new filtration. The first term of the Lagrangian filtration is the Lagrangian mapping class group, whose definition involves a handlebody bounded by Σ, and which contains the Torelli group. These constructions extend in a natural way to the monoid of homology cobordisms. Besides, D. Cheptea, K. Habiro and G. Massuyeau constructed a functorial extension of the LMO invariant, called the LMO functor, which takes values in a category of diagrams. In this paper we give a topological interpretation of the upper part of the tree reduction of the LMO functor in terms of the homomorphisms defined by J. Levine for the Lagrangian mapping class group. We also compare the Johnson filtration with the filtration introduced by J. Levine.

MSC classification

Primary: 57M27: Invariants of knots and 3-manifolds
Secondary: 57M05: Fundamental group, presentations, free differential calculus
Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 170 , Issue 2 , March 2021 , pp. 291 - 325
Copyright
© Cambridge Philosophical Society 2019

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bar–Natan, D., Garoufalidis, S., Rozansky, L. and Thurston, D. P.. The Aarhus integral of rational homology 3-spheres. I. A highly non trivial flat connection on S 3. Selecta Math. (N.S.) 8(3) (2002), 315–339.CrossRefGoogle Scholar
Bar–Natan, D., Garoufalidis, S., Rozansky, L. and Thurston, D. P.. The Aarhus integral of rational homology 3-spheres. II. Invariance and universality. Selecta Math. (N.S.) 8(3) (2002), 341–371.CrossRefGoogle Scholar
Broaddus, N., Farb, B. and Putman, A.. Irreducible Sp-representations and subgroup distortion in the mapping class group. Comment. Math. Helv. 86(3) (2011), 537–556.CrossRefGoogle Scholar
Cheptea, D., Habiro, K. and Massuyeau, G.. A functorial LMO invariant for Lagrangian cobordisms. Geom. Topol. 12(2) (2008), 1091–1170.CrossRefGoogle Scholar
Cochran, T. D.. Derivatives of links: Milnor’s concordance invariants and Massey’s products. Mem. Amer. Math. Soc. 84(427) (1990), x+73.Google Scholar
Cochran, T. D.. k-cobordism for links in S3. Trans. Amer. Math. Soc. 327(2) (1991), 641–654.Google Scholar
Conant, J., Schneiderman, R. and Teichner, P.. Milnor invariants and twisted Whitney towers. J. Topol. 7(1) (2014), 187–224.CrossRefGoogle Scholar
Garoufalidis, S. and Levine, J.. Tree-level invariants of three-manifolds, Massey products and the Johnson homomorphism. In Graphs and patterns in mathematics and theoretical physics, volume 73 of Proc. Sympos. Pure Math., pages 173–203 (Amer. Math. Soc., Providence, RI, 2005.CrossRefGoogle Scholar
Goussarov, M.. Finite type invariants and n-equivalence of 3-manifolds. C. R. Acad. Sci. Paris Sér. I Math. 329(6) (1999), 517–522.CrossRefGoogle Scholar
Goussarov, M. N.. Variations of knotted graphs. The geometric technique of n-equivalence. Algebra i Analiz 12(4) (2000), 79–125.Google Scholar
Griffiths, H. B.. Automorphisms of a 3-dimensional handlebody. Abh. Math. Sem. Univ. Hamburg 26 (1963/1964), 191–210.CrossRefGoogle Scholar
Habegger, N.. Milnor, Johnson and tree level perturbative invariants. Preprint (2000).Google Scholar
Habegger, N. and Lin, X. S.. The classification of links up to link-homotopy. J. Amer. Math. Soc. 3(2) (1990), 389–419.CrossRefGoogle Scholar
Habegger, N. and Lin, X. S.. On link concordance and Milnor’s $\overline {\mu}$invariants. Bull. London Math. Soc. 30(4) (1998), 419–428.CrossRefGoogle Scholar
Habegger, N. and Masbaum, G.. The Kontsevich integral and Milnor’s invariants. Topology 39(6) (2000), 1253–1289.CrossRefGoogle Scholar
Habiro, K.. Claspers and finite type invariants of links. Geom. Topol. 4 (2000), 1–83.CrossRefGoogle Scholar
Habiro, K. and Massuyeau, G.. From mapping class groups to monoids of homology cobordisms: a survey. In Handbook of Teichmüller theory. Volume III, volume 17 of IRMA Lect. Math. Theor. Phys., pages 465–529 (Eur. Math. Soc., Zürich, 2012).CrossRefGoogle Scholar
Johnson, D.. An abelian quotient of the mapping class group $\mathcal{I}_g$. Math. Ann. 249(3) (1980), 225–242.CrossRefGoogle Scholar
Johnson, D.. A survey of the Torelli group. In Low-dimensional topology (San Francisco, Calif., 1981), volume 20 of Contemp. Math., pages 165–179 (Amer. Math. Soc., Providence, RI, 1983).CrossRefGoogle Scholar
Johnson, D.. The structure of the Torelli group. III. The abelianisation of $\mathcal{T}$. Topology 24(2) (1985), 127–144.CrossRefGoogle Scholar
Le, T. T. Q., Murakami, J. and Ohtsuki, T.. On a universal perturbative invariant of 3-manifolds. Topology 37(3) (1998), 539–574.CrossRefGoogle Scholar
Levine, J.. Homology cylinders: an enlargement of the mapping class group. Algebr. Geom. Topol. 1 (2001), 243–270.CrossRefGoogle Scholar
Levine, J.. Addendum and correction to: “Homology cylinders: an enlargement of the mapping class group” [Algebr. Geom. Topol. 1 (2001), 243–270; MR1823501 (2002m:57020)]. Algebr. Geom. Topol. 2 (2002), 1197–1204.CrossRefGoogle Scholar
Levine, J.. Labeled binary planar trees and quasi-Lie algebras. Algebr. Geom. Topol. 6 (2006), 935–948.CrossRefGoogle Scholar
Levine, J.. The Lagrangian filtration of the mapping class group and finite-type invariants of homology spheres. Math. Proc. Camb. Phil. Soc. 141(2) (2006), 303–315.CrossRefGoogle Scholar
Massuyeau, G.. Infinitesimal Morita homomorphisms and the tree-level of the LMO invariant. Bull. Soc. Math. France 140(1) (2012), 101–161.CrossRefGoogle Scholar
Massuyeau, G. and Meilhan, J.B.. Equivalence relations for homology cylinders and the core of the Casson invariant. Trans. Amer. Math. Soc. 365(10) (2013), 5431–5502.CrossRefGoogle Scholar
Morita, S.. Casson’s invariant for homology 3-spheres and characteristic classes of surface bundles. I. Topology 28(3) (1989), 305–323.CrossRefGoogle Scholar
Morita, S.. On the structure of the Torelli group and the Casson invariant. Topology 30(4) 1991, 603–621.CrossRefGoogle Scholar
Morita, S.. Abelian quotients of subgroups of the mapping class group of surfaces. Duke Math. J. 70(3) (1993), 699–726.CrossRefGoogle Scholar
Morita, S.. Casson invariant, signature defect of framed manifolds and the secondary characteristic classes of surface bundles. J. Differential Geom. 47(3) (1997), 560–599.CrossRefGoogle Scholar
Oda, T.. A lower bound for the graded modules associated with the relative weight filtration on the teichmuller group. Preprint (1992).Google Scholar
Ohtsuki, T.. Quantum invariants, volume 29 of Series on Knots and Everything (World Scientific Publishing Co., Inc., River Edge, NJ, 2002). A study of knots, 3-manifolds, and their sets.CrossRefGoogle Scholar
Orr, K. E.. Homotopy invariants of links. Invent. Math. 95(2) (1989), 379–394.CrossRefGoogle Scholar
Sakasai, T.. Lagrangian mapping class groups from a group homological point of view. Algebr. Geom. Topol. 12(1) (2012), 267–291.CrossRefGoogle Scholar
Satoh, T.. On the Johnson homomorphisms of the mapping class groups of surfaces. In Handbook of group actions. Vol. I, volume 31 of Adv. Lect. Math. (ALM), pages 373–407 (Int. Press, Somerville, MA, 2015).Google Scholar
Stallings, J.. Homology and central series of groups. J. Algebra 2 (1965), 170–181.CrossRefGoogle Scholar
Suzuki, S.. On homeomorphisms of a 3-dimensional handlebody. Canad. J. Math. 29(1) (1977), 111–124.CrossRefGoogle Scholar