Article contents
Hausdorff dimension of level sets of generalized Takagi functions
Published online by Cambridge University Press: 19 June 2014
Abstract
This paper examines the Hausdorff dimension of the level sets f−1(y) of continuous functions of the form
 \begin{equation*}
f(x)=\sum_{n=0}^\infty 2^{-n}\omega_n(x)\phi(2^n x), \quad 0\leq x\leq 1,
\end{equation*}
\begin{equation*}
f(x)=\sum_{n=0}^\infty 2^{-n}\omega_n(x)\phi(2^n x), \quad 0\leq x\leq 1,
\end{equation*}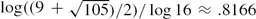 $\log ((9+\sqrt{105})/2)/\log 16\approx .8166$, but in case each ωn is constant, the largest possible dimension is 1/2. These results are extended to the intersection of the graph of f with lines of arbitrary integer slope. Furthermore, two natural models of choosing the signs ωn(x) at random are considered, and almost-sure results are obtained for the Hausdorff dimension of the zero set and the set of maximum points of f. The paper ends with a list of open problems.
$\log ((9+\sqrt{105})/2)/\log 16\approx .8166$, but in case each ωn is constant, the largest possible dimension is 1/2. These results are extended to the intersection of the graph of f with lines of arbitrary integer slope. Furthermore, two natural models of choosing the signs ωn(x) at random are considered, and almost-sure results are obtained for the Hausdorff dimension of the zero set and the set of maximum points of f. The paper ends with a list of open problems.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 157 , Issue 2 , September 2014 , pp. 253 - 278
- Copyright
- Copyright © Cambridge Philosophical Society 2014
References
REFERENCES
- 1
- Cited by


