Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-24T02:24:53.576Z Has data issue: false hasContentIssue false
  • English
  • Français

The Hankel transform of Whittaker's function Wk, m(z)

Published online by Cambridge University Press:  24 October 2008

A. Erdélyi
A. Erdélyi
Affiliation:
Brno, Czechoslovakia
Get access Rights & Permissions [Opens in a new window]

Extract

The Hankel transform of a function x−½νf(x) can be determined by the help of the following rule, obtained for ν = 0, 1 by B. van der Pol and K. Niessen(1), for positive integral values of ν by K. Niessen(2), and for arbitrary values of ν, [R(ν) > − 1], by F. Tricomi(3). If*

F(p) ≒ f(x),

then

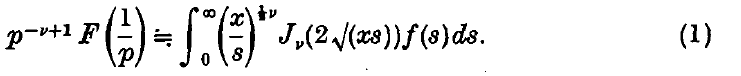
Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 34 , Issue 1 , January 1938 , pp. 28 - 29
Copyright
Copyright © Cambridge Philosophical Society 1938

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)van der Pol, B. and Niessen, K., Phil. Mag. 13 (1932), 537.CrossRefGoogle Scholar
(2)Niessen, K., Phil. Mag. 20 (1935), 977, equation (1).CrossRefGoogle Scholar
(3)Tricomi, F., Rend. dei Lincei, 22 (1935), 564, § 3.Google Scholar
(4)Whittaker, E. T. and Watson, G. N., Modern analysis, 4th ed. (Cambridge, 1927), chapter 16.Google Scholar
(5)Goldstein, S., Proc. London Math. Soc. (2), 34 (1932), 103, equation (80). See also Erdélyi A., Math. Zeitschrift, 42 (1936), 125, equation (2, 7).CrossRefGoogle Scholar
(6)Varma, R. S., Proc. Cambridge Phil. Soc. 33 (1937), 210.CrossRefGoogle Scholar