Hostname: page-component-745bb68f8f-l4dxg Total loading time: 0 Render date: 2025-01-12T08:44:49.275Z Has data issue: false hasContentIssue false
  • English
  • Français

The existence of Bartlett-Rajalakshman goodness of fit G-tests for multivariate autoregressive processes with finitely dependent residuals

Published online by Cambridge University Press:  24 October 2008

A. M. Walker  and
D. V. Lindley
A. M. Walker
Affiliation:
Unit of BiometryOxford
Get access Rights & Permissions [Opens in a new window]

Extract

Bartlett and Rajalakshman (3) have derived two types of large sample goodness of fit tests for the hypothesis that a multivariate (or vector) time series {X(t)}, t = 0, ± 1, ± 2, …, is generated by a linear autoregressive process. This may be defined as the stationary solution of an equation of the form

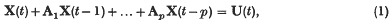
where the Ai are square matrices such that the roots of the determinantal equation ‖ zp + A1zp−1 + … + Ap ‖ = 0 have moduli less than unity, and {U(t)} is a sequence of independent random vector variables with a common distribution.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 54 , Issue 2 , April 1958 , pp. 225 - 232
Copyright
Copyright © Cambridge Philosophical Society 1958

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Bartlett, M. S.An introduction to stochastic processes (Cambridge, 1955).Google Scholar
(2)Bartlett, M. S. and Diananda, P. H.J. Roy. Statist. Soc. B, 12 (1950), 108–15.Google Scholar
(3)Bartlett, M. S. and Rajalakshman, D. V.J. Roy. Statist. Soc. B, 15 (1953), 107–24.Google Scholar
(4)Quenouille, M. H.J. Roy. Statist. Soc. A, 110 (1947), 123–9.CrossRefGoogle Scholar
(5)Quenouille, M. H.The analysis of multiple time series (London, 1957).Google Scholar
(6)Walker, A. M.J. Roy. Statist. Soc. B, 14 (1952), 117–34.Google Scholar
(7)Walker, A. M. and Young, A.M. Not. Roy. Astr. Soc. 115 (1955), 443–59.CrossRefGoogle Scholar
(8)Whittle, P.J. Roy. Statist. Soc. B, 15 (1953), 125–39.Google Scholar
(9)Wold, H.J. Roy. Statist. Soc. B, 11 (1949), 297–305.Google Scholar