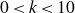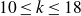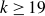Differential forms on universal K3 surfaces

Abstract

We give a vanishing and classification result for holomorphic differential forms on smooth projective models of the moduli spaces of pointed K3 surfaces. We prove that there is no nonzero holomorphic k-form for $0<k<10$ and for even $k>19$. In the remaining cases, we give an isomorphism between the space of holomorphic k-forms with that of vector-valued modular forms ($10\leq k \leq 18$) or scalar-valued cusp forms (odd $k\geq 19$) for the modular group. These results are in fact proved in the generality of lattice-polarisation.

1. Introduction

Let ${{\mathcal{F}_{g,n}}}$ be the moduli space of n-pointed K3 surfaces of genus $g>2$ , i.e., primitively polarised of degree $2g-2$ . It is a quasi-projective variety of dimension $19+2n$ with a natural morphism ${{\mathcal{F}_{g,n}}}\to {{\mathcal{F}_{g}}}$ to the moduli space ${{\mathcal{F}_{g}}}$ of K3 surfaces of genus g, which is generically a $K3^{n}$ -fibration. In this paper we study holomorphic differential k-forms on a smooth projective model of ${{\mathcal{F}_{g,n}}}$ . They do not depend on the choice of a smooth projective model, and thus are fundamental birational invariants of ${{\mathcal{F}_{g,n}}}$ . We prove a vanishing result for about half of the values of the degree k, and for the remaining degrees give a correspondence with modular forms on the period domain.

Our main result is stated as follows.

Theorem 1·1. Let ${{\bar{\mathcal{F}}_{g,n}}}$ be a smooth projective model of ${{\mathcal{F}_{g,n}}}$ with $g>2$ . Then we have a natural isomorphism:

(1·1) \begin{equation} H^{0}({{\bar{\mathcal{F}}_{g,n}}}, \Omega^{k}) \simeq\begin{cases}0 & \: \: 0<k\leq 9 \\ M_{\wedge^{k},k}({{\Gamma_{g}}}) & \: \: 10 \leq k \leq 18 \\ 0 & \: \: k>19, \: k\in 2{{\mathbb{Z}}} \\ S_{19+m}({{\Gamma_{g}}}, \det)\otimes {{\mathbb{C}}}\mathcal{S}_{n,m} & \: \: k=19+2m, \: 0\leq m \leq n\end{cases}. \end{equation}

Here ${{\Gamma_{g}}}$ is the modular group for K3 surfaces of genus g, which is defined as the kernel of $\mathrm{O}^{+}(L_{g})\to \mathrm{O}(L_{g}^{\vee}/L_{g})$ where $L_{g}=2U\oplus 2E_{8}\oplus \langle 2-2g \rangle$ is the period lattice of K3 surfaces of genus g. In the second case, $M_{\wedge^{k},k}({{\Gamma_{g}}})$ stands for the space of vector-valued modular forms of weight $(\wedge^{k},k)$ for ${{\Gamma_{g}}}$ (see [ 4 ]). In the last case, $S_{19+m}({{\Gamma_{g}}}, \det)$ stands for the space of scalar-valued cusp forms of weight $19+m$ and determinant character for ${{\Gamma_{g}}}$ , and $\mathcal{S}_{n,m}$ stands for the right quotient $\mathfrak{S}_{n}/(\mathfrak{S}_{m}\times \mathfrak{S}_{n-m})$ , which is a left $\mathfrak{S}_{n}$ -set. Theorem 1·1 is actually formulated and proved in the generality of lattice-polarisation (Theorem 2·6).

In the case of the top degree $k=19+2n$ , namely for canonical forms, the isomorphism (1·1) is proved in [ 2 ]. Theorem 1·1 is the extension of this result to all degrees $k<19+2n$ . The spaces in the right-hand side of (1·1) can also be geometrically explained as follows. In the case $k\leq 18$ , $M_{\wedge^{k},k}({{\Gamma_{g}}})$ is identified with the space of holomorphic k-forms on a smooth projective model of ${{\mathcal{F}_{g}}}$ , pulled back by ${{\mathcal{F}_{g,n}}}\to {{\mathcal{F}_{g}}}$ . In the case $k=19+2m$ , $S_{19+m}({{\Gamma_{g}}}, \det)$ is identified with the space of canonical forms on $\bar{\mathcal{F}}_{g,m}$ , and the tensor product $S_{19+m}({{\Gamma_{g}}}, \det)\otimes {{\mathbb{C}}}\mathcal{S}_{n,m}$ is the direct sum of pullback of such canonical forms by various projections ${{\mathcal{F}_{g,n}}}\to \mathcal{F}_{g,m}$ . Therefore Theorem 1·1 can be understood as a kind of classification result which says that except for canonical forms, there are essentially no new differential forms on the tower $({{\mathcal{F}_{g,n}}})_{n}$ of moduli spaces. In fact, this is how the proof proceeds.

The space $S_{l}({{\Gamma_{g}}}, \det)$ is nonzero for every sufficiently large l, so the space $H^{0}({{\bar{\mathcal{F}}_{g,n}}}, \Omega^{k})$ for odd $k\geq 19$ is typically nonzero (at least when k is large). On the other hand, it is not clear at present whether $M_{\wedge^{k},k}({{\Gamma_{g}}})\ne 0$ or not in the range $10\leq k \leq 18$ . This is a subject of study in the theory of vector-valued orthogonal modular forms.

The isomorphism (1·1) in the case $k=19+2m$ is an $\mathfrak{S}_{n}$ -equivariant isomorphism, where $\mathfrak{S}_{n}$ acts on $H^{0}({{\bar{\mathcal{F}}_{g,n}}}, \Omega^{k})$ by its permutation action on ${{\mathcal{F}_{g,n}}}$ , while it acts on $S_{19+m}({{\Gamma_{g}}}, \det)\otimes {{\mathbb{C}}}\mathcal{S}_{n,m}$ by its natural left action on $\mathcal{S}_{n,m}$ . Therefore, taking the $\mathfrak{S}_{n}$ -invariant part, we obtain the following simpler result for the unordered pointed moduli space ${{\mathcal{F}_{g,n}}}/\mathfrak{S}_{n}$ , which is birationally a $K3^{[n]}$ -fibration over ${{\mathcal{F}_{g}}}$ .

Corollary 1·2. Let $\overline{{{\mathcal{F}_{g,n}}}/\mathfrak{S}_{n}}$ be a smooth projective model of ${{\mathcal{F}_{g,n}}}/\mathfrak{S}_{n}$ . Then we have a natural isomorphism:

\begin{equation*}H^{0}(\overline{{{\mathcal{F}_{g,n}}}/\mathfrak{S}_{n}}, \Omega^{k}) \simeq\begin{cases}0 & \: \: 0<k\leq 9 \\ M_{\wedge^{k},k}({{\Gamma_{g}}}) & \: \: 10\leq k \leq 18 \\ 0 & \: \: k>19, \: k\in 2{{\mathbb{Z}}} \\ S_{19+m}({{\Gamma_{g}}}, \det) & \: \: k=19+2m, \: 0 \leq m \leq n\end{cases}.\end{equation*}

The universal K3 surface $\mathcal{F}_{g,1}$ is an analogue of elliptic modular surfaces ([ 6 ]), and the moduli spaces ${{\mathcal{F}_{g,n}}}$ for general n are analogues of the so-called Kuga varieties over modular curves ([ 7 ]). Starting with the case of elliptic modular surfaces [ 6 ], holomorphic differential forms on the Kuga varieties have been described in terms of elliptic modular forms: [ 7 ] for canonical forms, and [ 1 ] for the case of lower degrees (somewhat implicitly). Theorem 1·1 can be regarded as a K3 version of these results.

As a final remark, in view of the analogy between universal K3 surfaces and elliptic modular surfaces, invoking the classical fact that elliptic modular surfaces have maximal Picard number ([ 6 ]) now raises the question if $H^{k,0}({{\bar{\mathcal{F}}_{g,n}}})\oplus H^{0,k}({{\bar{\mathcal{F}}_{g,n}}})$ is a sub ${{\mathbb{Q}}}$ -Hodge structure of $H^{k}({{\bar{\mathcal{F}}_{g,n}}}, {{\mathbb{C}}})$ . This is independent of the choice of a smooth projective model ${{\bar{\mathcal{F}}_{g,n}}}$ .

The rest of this paper is devoted to the proof of Theorem 1·1. In Section 2·1 we compute a part of the holomorphic Leray spectral sequence associated to a certain type of $K3^{n}$ -fibration. This is the main step of the proof. In Section 2·2 we study differential forms on a compactification of such a fibration. In Section 2·3 we deduce (a generalised version of) Theorem 1·1 by combining the result of Section 2·2 with some results from [ 2–5 ]. Sometimes we drop the subscript X from the notation $\Omega_{X}^{k}$ when the variety X is clear from the context.

2. Proof

2·1. Holomorphic Leray spectral sequence

Let $\pi\colon X\to B$ be a smooth family of K3 surfaces over a smooth connected base B. In this subsection X and B may be analytic. We put the following assumption:

Condition 2·1. In a neighbourhood of every point of B, the period map is an embedding.

This is equivalent to the condition that the differential of the period map

\begin{equation*}T_{b}B \to \mathrm{Hom}(H^{2,0}(X_{b}), H^{1,1}(X_{b}))\end{equation*}

is injective for every $b\in B$ , where $X_{b}$ is the fiber of $\pi$ over b.

For a natural number $n>0$ we denote by $X_{n}=X\times_{B}\cdots \times_{B}X$ the n-fold fiber product of X over B, and let $\pi_{n}\colon X_{n}\to B$ be the projection. We denote by $\Omega_{\pi_{n}}$ the relative cotangent bundle of $\pi_{n}$ , and $\Omega_{\pi_{n}}^{p}=\wedge^{p}\Omega_{\pi_{n}}$ for $p\geq 0$ as usual.

Proposition 2·2. Let $\pi\colon X\to B$ be a K3 fibration satisfying Condition 2·1. Then we have a natural isomorphism:

\begin{equation*}(\pi_{n})_{\ast}\Omega_{X_{n}}^{k} \simeq\begin{cases}\Omega_{B}^{k} & \; \; k\leq \dim B \\ 0 & \; \; k>\dim B, \: \: k\not \equiv \dim B \: \: \mathrm{mod} \; 2 \\ K_{B}\otimes (\pi_{n})_{\ast}\Omega_{\pi_{n}}^{2m} & \; \; k=\dim B+2m, \: 0\leq m \leq n\end{cases}.\end{equation*}

This assertion amounts to a partial degeneration of the holomorphic Leray spectral sequence. Recall ([ 8 , section 5·2]) that $\Omega_{X_{n}}^k$ has the holomorphic Leray filtration $L^{\bullet}\Omega_{X_{n}}^k$ defined by

\begin{equation*}L^{l}\Omega_{X_{n}}^k= \pi_{n}^{\ast}\Omega_{B}^{l}\wedge \Omega_{X_{n}}^{k-l},\end{equation*}

whose graded quotients are naturally isomorphic to

\begin{equation*}\mathrm{Gr}_{L}^{l}\Omega_{X_{n}}^{k} = \pi_{n}^{\ast}\Omega_{B}^{l} \otimes \Omega_{\pi_{n}}^{k-l}.\end{equation*}

This filtration induces the holomorphic Leray spectral sequence

\begin{equation*}(E_{r}^{l,q}, d_{r}) \: \: \: \Rightarrow \: \: \: E_{\infty}^{l+q} = R^{l+q}(\pi_{n})_{\ast}\Omega_{X_{n}}^{k}\end{equation*}

which converges to the filtration

\begin{equation*}L^{l}R^{l+q}(\pi_{n})_{\ast}\Omega_{X_{n}}^{k} \: = \:\mathrm{Im}(R^{l+q}(\pi_{n})_{\ast}L^{l}\Omega_{X_{n}}^{k} \to R^{l+q}(\pi_{n})_{\ast}\Omega_{X_{n}}^{k}).\end{equation*}

By [ 8 , proposition 5·9], the $E_{1}$ page coincides with the collection of the Koszul complexes associated to the variation of Hodge structures for $\pi_{n}$ :

(2·1) \begin{equation}(E_{1}^{l,q}, d_{1}) = (\mathcal{H}^{k-l,l+q}\otimes \Omega_{B}^{l}, \bar{\nabla}).\end{equation}

Here $\mathcal{H}^{\ast, \ast}$ are the Hodge bundles associated to the fibration $\pi_{n}\colon X_{n}\to B$ , and

\begin{equation*}\bar{\nabla} : \mathcal{H}^{\ast, \ast}\otimes \Omega_{B}^{\ast} \to \mathcal{H}^{\ast-1, \ast+1}\otimes \Omega_{B}^{\ast+1}\end{equation*}

are the differentials in the Koszul complexes (see [ 8 , section 5·1·3]). For degree reasons, the range of (l, q) in the $E_{1}$ page satisfies the inequalities

\begin{equation*}0\leq l \leq \dim B, \quad 0\leq k-l \leq 2n, \quad 0\leq l+q \leq 2n.\end{equation*}

The first two can be unified:

(2·2) \begin{equation}\max\!(0, k-2n) \leq l \leq \min\!(\!\dim B, k), \quad 0\leq l+q \leq 2n.\end{equation}

We calculate the $E_{1}$ to $E_{2}$ pages on the edge line $l+q=0$ .

Lemma 2·3. The following holds:

1. (1) $E_{1}^{l,-l}=0$ when $l\leq \min\!(\!\dim B, k)$ with $l\not\equiv k$ mod 2;

2. (2) $E_{2}^{l,-l}=0$ when $l< \min\!(\!\dim B, k)$ ;

3. (3) For $l_{0} = \min\!(\!\dim B, k)$ we have $E_{1}^{l_{0},-l_{0}}=E_{2}^{l_{0},-l_{0}}= \cdots = E_{\infty}^{l_{0},-l_{0}}$ .

Proof. By (2·1), we have $E_{1}^{l,-l}=\mathcal{H}^{k-l,0}\otimes \Omega_{B}^{l}$ . By the Künneth formula, the fiber of $\mathcal{H}^{k-l,0}$ over a point $b\in B$ is identified with

(2·3) \begin{equation}H^{k-l,0}(X_{b}^{n}) = \bigoplus_{(p_{1}, \cdots, p_{n})} H^{p_{1},0}(X_{b}) \otimes \cdots \otimes H^{p_{n},0}(X_{b}),\end{equation}

where $(p_{1}, \cdots, p_{n})$ ranges over all indices with $\sum_{i}p_{i}=k-l$ and $0\leq p_{i} \leq 2$ .

(1) When $k-l$ is odd, every index $(p_{1}, \cdots, p_{n})$ in (2·3) must contain a component $p_{i}=1$ . Since $H^{1,0}(X_b)=0$ , we see that $H^{k-l,0}(X_{b}^{n})=0$ . Therefore $\mathcal{H}^{k-l,0}=0$ when $k-l$ is odd.

(3) Let $l_{0} = \min\!(\!\dim B, k)$ . By the range (2·2) of (l, q), we see that for every $r\geq 1$ the source of $d_{r}$ that hits $E_{r}^{l_{0}, -l_{0}}$ is zero, and the target of $d_{r}$ that starts from $E_{r}^{l_{0}, -l_{0}}$ is also zero. This proves our assertion.

(2) Let $l < \min\!(\!\dim B, k)$ . In view of (1), we may assume that $l=k-2m$ for some $m>0$ . By (2·2), the source of $d_{1}$ that hits $E_{1}^{l,-l}$ is zero. We shall show that $d_{1}\colon E_{1}^{l,-l}\to E_{1}^{l+1,-l}$ is injective. By (2·1), this morphism is identified with

(2·4) \begin{equation}\bar{\nabla} : \mathcal{H}^{2m,0}\otimes \Omega_{B}^{l} \to \mathcal{H}^{2m-1,1}\otimes \Omega_{B}^{l+1}.\end{equation}

By the Künneth formula as in (2·3), the fibers of the Hodge bundles $\mathcal{H}^{2m,0}$ , $\mathcal{H}^{2m-1,1}$ over $b\in B$ are respectively identified with

(2·5) \begin{equation}H^{2m,0}(X_{b}^{n}) = \bigoplus_{|\sigma|=m} H^{2,0}(X_{b})^{\otimes \sigma},\end{equation}

(2·6) \begin{eqnarray}H^{2m-1,1}(X_{b}^{n})& = & \bigoplus_{|\sigma'|=m-1} \bigoplus_{i\not\in \sigma'} H^{2,0}(X_{b})^{\otimes \sigma'}\otimes H^{1,1}(X_{b}) \\ & = & \bigoplus_{|\sigma|=m} \bigoplus_{i\in \sigma} H^{2,0}(X_{b})^{\otimes \sigma-\{ i \}}\otimes H^{1,1}(X_{b}). \nonumber\end{eqnarray}

In (2·5), $\sigma$ ranges over all subsets of $\{ 1, \cdots, n\}$ consisting of m elements, and $H^{2,0}(X_{b})^{\otimes \sigma}$ stands for the tensor product of $H^{2,0}(X_{b})$ for the jth factors $X_{b}$ of $X_{b}^{n}$ over all $j\in \sigma$ . The notations $\sigma', \sigma$ in (2·6) are similar, and $H^{1,1}(X_{b})$ in (2·6) is the $H^{1,1}$ of the ith factor $X_{b}$ of $X_{b}^{n}$ .

Let us write $V=H^{2,0}(X_{b})$ and $W=(T_{b}B)^{\vee}$ for simplicity. The homomorphism (2·4) over $b\in B$ is written as

(2·7) \begin{equation}\bigoplus_{|\sigma|=m} \left( V^{\otimes \sigma}\otimes \wedge^{l}W \to\bigoplus_{i\in \sigma} V^{\otimes \sigma - \{ i\}}\otimes H^{1,1}(X_{b})\otimes \wedge^{l+1}W \right).\end{equation}

By [ 8 , lemma 5·8], the $(\sigma, i)$ -component

(2·8) \begin{equation}V^{\otimes \sigma}\otimes \wedge^{l}W \to V^{\otimes \sigma - \{ i\}}\otimes H^{1,1}(X_{b})\otimes \wedge^{l+1}W\end{equation}

factorises as

\begin{eqnarray*}V^{\otimes \sigma}\otimes \wedge^{l}W & \to &V^{\otimes \sigma - \{ i\}}\otimes H^{1,1}(X_{b}) \otimes W \otimes \wedge^{l}W \\ & \to & V^{\otimes \sigma - \{ i\}}\otimes H^{1,1}(X_{b})\otimes \wedge^{l+1}W,\end{eqnarray*}

where the first map is induced by the adjunction $V\to H^{1,1}(X_b)\otimes W$ of the differential of the period map for the ith factor $X_{b}$ , and the second map is induced by the wedge product $W \otimes \wedge^{l}W \to \wedge^{l+1}W$ . By linear algebra, this composition can also be decomposed as

(2·9) \begin{eqnarray}V^{\otimes \sigma}\otimes \wedge^{l}W & \to &V^{\otimes \sigma - \{ i\}}\otimes V \otimes W^{\vee} \otimes \wedge^{l+1}W \\ & \to & V^{\otimes \sigma - \{ i\}}\otimes H^{1,1}(X_{b})\otimes \wedge^{l+1}W, \nonumber\end{eqnarray}

where the first map is induced by the adjunction $\wedge^{l}W \to W^{\vee} \otimes \wedge^{l+1}W$ of the wedge product, and the second map is induced by the adjunction $V\otimes W^{\vee}\to H^{1,1}(X_{b})$ of the differential of the period map. By our initial Condition 2·1, the second map of (2·9) is injective. Moreover, since $l+1\leq \dim W$ by our assumption, the wedge product $\wedge^{l}W\times W \to \wedge^{l+1}W$ is nondegenerate, so its adjunction $\wedge^{l}W \to W^{\vee} \otimes \wedge^{l+1}W$ is injective. Thus the first map of (2·9) is also injective. It follows that (2·8) is injective. Since the map (2·7) is the direct sum of its $(\sigma, i)$ -components, it is injective. This finishes the proof of Lemma 2·3.

We can now complete the proof of Proposition 2·2.

Proof of Proposition 2·2. By Lemma 2·3 (2), we have $E_{\infty}^{l,-l}=0$ when $l<l_{0}=\min\!(\!\dim B, k)$ . Together with Lemma 2·3 (3), we obtain

\begin{equation*}(\pi_{n})_{\ast}\Omega_{X_{n}}^{k} = E_{\infty}^{0} = E_{\infty}^{l_{0}, -l_{0}} = E_{1}^{l_{0}, -l_{0}}.\end{equation*}

When $k\leq \dim B$ , we have $l_{0}=k$ , and $E_{1}^{l_{0}, -l_{0}}=\Omega_{B}^{k}$ by (2·1). When $k> \dim B$ , we have $l_{0}= \dim B$ , and $E_{1}^{l_{0}, -l_{0}}=\mathcal{H}^{k-\dim B, 0}\otimes K_{B}$ by (2·1). When $k-\dim B$ is odd, this vanishes by Lemma 2·3 (1).

In the case $k=\dim B + 2m$ , the vector bundle $\mathcal{H}^{2m,0}\otimes K_{B}=(\pi_{n})_{\ast}\Omega_{\pi_{n}}^{2m}\otimes K_{B}$ can be written more specifically as follows. For a subset $\sigma$ of $\{ 1, \cdots, n \}$ with cardinality $| \sigma |=m$ , we denote by $X_{\sigma}\simeq X_{m}$ the fiber product of the ith factors $X\to B$ of $X_{n}\to B$ over all $i\in \sigma$ . We denote by

\begin{equation*}X_{n} \stackrel{\pi_{\sigma}}{\to} X_{\sigma} \stackrel{\pi^{\sigma}}{\to} B\end{equation*}

the natural projections. The Künneth formula (2·5) says that

\begin{equation*}(\pi_{n})_{\ast}\Omega_{\pi_{n}}^{2m} \simeq\bigoplus_{|\sigma|=m} \pi^{\sigma}_{\ast}K_{\pi^{\sigma}}.\end{equation*}

Combining this with the isomorphism

(2·10) \begin{equation}\pi^{\sigma}_{\ast}K_{X_{\sigma}}\simeq K_{B}\otimes \pi^{\sigma}_{\ast}K_{\pi^{\sigma}}\end{equation}

for each $X_{\sigma}$ , we can rewrite the isomorphism in the last case of Proposition 2·2 as

(2·11) \begin{equation}(\pi_{n})_{\ast}\Omega_{X_{n}}^{\dim B+2m} \simeq\bigoplus_{|\sigma|=m} \pi^{\sigma}_{\ast}K_{X_{\sigma}}.\end{equation}

2·2. Extension over compactification

Let $\pi\colon X\to B$ be a K3 fibration as in Section 2·1. We now assume that X, B are quasi-projective and $\pi$ is a morphism of algebraic varieties. We take smooth projective compactifications of $X_{n}, X_{\sigma}, B$ and denote them by $\bar{X}_{n}, \bar{X}_{\sigma}, \bar{B}$ respectively.

Proposition 2·4. We have

\begin{equation*}H^{0}(\bar{X}_{n}, \Omega^{k}) \simeq\begin{cases}H^{0}(\bar{B}, \Omega^{k}) & \: \: k\leq \dim B \\ 0 & \: \: k>\dim B, \: \: k\not\equiv \dim B \: \: \mathrm{mod} \; 2 \\ \oplus_{\sigma}H^{0}(\bar{X}_{\sigma}, K_{\bar{X}_{\sigma}}) & \: \: k=\dim B+2m, \: 0\leq m \leq n\end{cases}\end{equation*}

In the last case, $\sigma$ ranges over all subsets of $\{ 1, \cdots, n \}$ with $|\sigma|=m$ . The isomorphism in the first case is given by the pullback by $\pi_{n}\colon X_{n}\to B$ , and the isomorphism in the last case is given by the direct sum of the pullbacks by $\pi_{\sigma}\colon X_{n}\to X_{\sigma}$ for all $\sigma$ .

Proof. The assertion in the case $k>\dim B$ with $k\not\equiv \dim B$ mod 2 follows directly from the second case of Proposition 2·2. Next we consider the case $k\leq \dim B$ . We may assume that $\pi_{n}\colon X_{n}\to B$ extends to a surjective morphism $\bar{X}_{n}\to \bar{B}$ . Let $\omega$ be a holomorphic k-form on $\bar{X}_{n}$ . By the first case of Proposition 2·2, we have $\omega|_{X_{n}}=\pi_{n}^{\ast}\omega_{B}$ for a holomorphic k-form $\omega_{B}$ on B. Since $\omega$ is holomorphic over $\bar{X}_{n}$ , $\omega_{B}$ is holomorphic over $\bar{B}$ as well by a standard property of holomorphic differential forms. (Otherwise $\omega$ must have pole at the divisors of $\bar{X}_{n}$ dominating the divisors of $\bar{B}$ where $\omega_{B}$ has pole.) Therefore the pullback $H^{0}(\bar{B}, \Omega^{k})\to H^{0}(\bar{X}_{n}, \Omega^{k})$ is surjective.

Finally, we consider the case $k=\dim B+2m$ , $0\leq m \leq n$ . Let $\omega$ be a holomorphic k-form on $\bar{X}_{n}$ . By (2·11), we can uniquely write $\omega|_{X_{n}}=\sum_{\sigma}\pi_{\sigma}^{\ast}\omega_{\sigma}$ for some canonical forms $\omega_{\sigma}$ on $X_{\sigma}$ .

Claim 2.5. For each $\sigma$ , $\omega_{\sigma}$ is holomorphic over $\bar{X}_{\sigma}$ .

Proof. We identify $X_{n}$ with the fiber product $X_{\sigma}\times_{B}X_{\tau}$ where $\tau=\{ 1, \cdots, n\} - \sigma$ is the complement of $\sigma$ . We may assume that this fiber product diagram extends to a commutative diagram of surjective morphisms

between smooth projective models. We take an irreducible subvariety $\tilde{B}\subset \bar{X}_{\tau}$ such that $\tilde{B}\to \bar{B}$ is surjective and generically finite. Then $\pi_{\tau}^{-1}(\tilde{B})\subset \bar{X}_{n}$ has a unique irreducible component dominating $\tilde{B}$ . We take its desingularisation and denote it by Y. By construction $\pi_{\sigma}|_{Y} \colon Y\to \bar{X}_{\sigma}$ is dominant (and so surjective) and generically finite. On the other hand, for any $\sigma'\ne \sigma$ with $|\sigma'|=m$ , the projection $\pi_{\sigma'}|_{Y} \colon Y\dashrightarrow X_{\sigma'}$ is not dominant. Indeed, such $\sigma'$ contains at least one component $i\in \tau$ , so if $Y\dashrightarrow X_{\sigma'}$ was dominant, then the ith projection $Y\dashrightarrow X$ would be also dominant, which is absurd because it factorises as $Y\to \tilde{B}\subset \bar{X}_{\tau}\dashrightarrow X$ .

We pullback the differential form $\omega=\pi_{\sigma}^{\ast}\omega_{\sigma}+\sum_{\sigma'\ne \sigma}\pi_{\sigma'}^{\ast}\omega_{\sigma'}$ to Y and denote it by $\omega|_{Y}$ . Since $\omega$ is holomorphic over $\bar{X}_{n}$ , $\omega|_{Y}$ is holomorphic over Y. Since $\pi_{\sigma'}^{\ast}\omega_{\sigma'}|_{Y}$ is the pullback of the canonical form $\omega_{\sigma'}$ on $X_{\sigma'}$ by the non-dominant map $Y \dashrightarrow X_{\sigma'}$ , it vanishes identically. Hence $\pi_{\sigma}^{\ast}\omega_{\sigma}|_{Y}=\omega|_{Y}$ is holomorphic over Y. Since $\pi_{\sigma}|_{Y}\colon Y \to \bar{X}_{\sigma}$ is surjective, this implies that $\omega_{\sigma}$ is holomorphic over $\bar{X}_{\sigma}$ as before.

The above argument will be clear if we consider over the generic point $\eta$ of B: we restrict $\omega$ to the fiber of $(X_{\eta})^{n}\to (X_{\eta})^{\tau}$ over the geometric point $\tilde{B}$ of $(X_{\eta})^{\tau}$ over $\eta$ .

By Claim 2.5, the pullback

\begin{equation*}(\pi_{\sigma}^{\ast})_{\sigma} :\bigoplus_{|\sigma|=m} H^{0}(\bar{X}_{\sigma}, K_{\bar{X}_{\sigma}}) \to H^{0}(\bar{X}_{n}, \Omega^{\dim B + 2m})\end{equation*}

is surjective. It is also injective as implied by (2·11). This proves Proposition 2·4.

2·3. Universal K3 surface

Now we prove Theorem 1·1, in the generality of lattice-polarisation. Let L be an even lattice of signature (2, d) which can be embedded as a primitive sublattice of the K3 lattice $3U\oplus 2E_{8}$ . We denote by

\begin{equation*}\mathcal{D} = \{ \: {{\mathbb{C}}} \omega \in {{{\mathbb P}}}L_{{{\mathbb{C}}}} \: | \: (\omega, \omega)=0, (\omega, \bar{\omega})>0 \: \}^{+}\end{equation*}

the Hermitian symmetric domain associated to L, where $+$ means a connected component.

Let $\pi\colon X\to B$ be a smooth projective family of K3 surfaces over a smooth quasi-projective connected base B. We say ([ 3 ]) that the family $\pi\colon X\to B$ is lattice-polarised with period lattice L if there exists a sub local system $\Lambda$ of $R^{2}\pi_{\ast}{{\mathbb{Z}}}$ such that each fiber $\Lambda_{b}$ is a hyperbolic sublattice of the Néron-Severi lattice $NS(X_{b})$ and the fibers of the orthogonal complement $\Lambda^{\perp}$ are isometric to L. Then we have a period map

\begin{equation*}\mathcal{P} \,:\, B \to {{\Gamma}}\backslash \mathcal{D}\end{equation*}

for some finite-index subgroup ${{\Gamma}}$ of $\mathrm{O}^{+}(L)$ . By Borel’s extension theorem, $\mathcal{P}$ is a morphism of algebraic varieties.

Let us put the assumption

(2·12) \begin{equation}\mathcal{P} \; \mathrm{is\ birational\ and} \: -\mathrm{id}\not\in {{\Gamma}}.\end{equation}

For such a family $\pi\colon X\to B$ , if we shrink B as necessary, then $\mathcal{P}$ is an open immersion and Condition 2·1 is satisfied. For example, the universal K3 surface $\mathcal{F}_{g,1}\to {{\mathcal{F}_{g}}}$ for $g>2$ restricted over a Zariski open set of ${{\mathcal{F}_{g}}}$ satisfies this assumption with $L=L_{g}$ and ${{\Gamma}}={{\Gamma_{g}}}$ (see Section 1 for these notations).

As in Section 1, we denote by $M_{\wedge^{k},k}({{\Gamma}})$ the space of vector-valued modular forms of weight $(\wedge^{k},k)$ for ${{\Gamma}}$ , $S_{l}({{\Gamma}}, \det)$ the space of scalar-valued cusp forms of weight l and character $\det$ for ${{\Gamma}}$ , and $\mathcal{S}_{n,m}=\mathfrak{S}_{n}/(\mathfrak{S}_{m}\times \mathfrak{S}_{n-m})$ .

Theorem 2·6. Let $\pi\colon X\to B$ be a lattice-polarised K3 family with period lattice L of signature (2, d) with $d\geq 3$ and monodromy group ${{\Gamma}}$ satisfying (2·12). Then we have an $\mathfrak{S}_{n}$ -equivariant isomorphism

\begin{equation*}H^{0}(\bar{X}_{n}, \Omega^{k}) \simeq\begin{cases}0 & \: \: 0<k< d/2 \\ M_{\wedge^{k},k}({{\Gamma}}) & \: \: d/2 \leq k < d \\ 0 & \: \: k>d, \: k-d\not\in 2{{\mathbb{Z}}} \\ S_{d+m}({{\Gamma}}, \det)\otimes {{\mathbb{C}}}\mathcal{S}_{n,m} & \: \: k=d+2m, \: 0\leq m \leq n\end{cases}.\end{equation*}

Proof. When $k\leq d$ , we have $H^{0}(\bar{X}_{n}, \Omega^{k}) \simeq H^{0}(\bar{B}, \Omega^{k})$ by Proposition 2·4. Then $\bar{B}$ is a smooth projective model of the modular variety ${{\Gamma}}\backslash \mathcal{D}$ . By a theorem of Pommerening [ 5 ], the space $H^{0}(\bar{B}, \Omega^{k})$ for $k<d$ is isomorphic to the space of ${{\Gamma}}$ -invariant holomorphic k-forms on $\mathcal{D}$ , which in turn is identified with the space $M_{\wedge^{k},k}({{\Gamma}})$ of vector-valued modular forms of weight $(\wedge^{k},k)$ for ${{\Gamma}}$ (see [ 4 ]). The vanishing of this space in $0<k<d/2$ is proved in [ 4 , theorem 1·2] in the case when L has Witt index 2, and in [ 4 , theorem 1·5 (1)] in the case when L has Witt index $\leq 1$ .

The vanishing in the case $k>d$ with $k\not\equiv d$ mod 2 follows from Proposition 2·4. Finally, we consider the case $k=d+2m$ , $0\leq m \leq n$ . By Proposition 2·4, we have a natural $\mathfrak{S}_{n}$ -equivariant isomorphism

\begin{equation*}H^{0}(\bar{X}_{n}, \Omega^{d+2m}) \simeq\bigoplus_{|\sigma|=m} H^{0}(\bar{X}_{\sigma}, K_{\bar{X}_{\sigma}}),\end{equation*}

where $\mathfrak{S}_{n}$ permutes the subsets $\sigma$ of $\{ 1, \cdots, n \}$ . Here note that the stabiliser of each $\sigma$ acts on $H^{0}(\bar{X}_{\sigma}, K_{\bar{X}_{\sigma}})$ trivially by (2·10). Therefore, as an $\mathfrak{S}_{n}$ -representation, the right-hand side can be written as

\begin{equation*}H^{0}(\bar{X}_{m}, K_{\bar{X}_{m}}) \otimes \left( \bigoplus_{|\sigma|=m}{{\mathbb{C}}}\sigma \right)\simeq H^{0}(\bar{X}_{m}, K_{\bar{X}_{m}}) \otimes {{\mathbb{C}}}\mathcal{S}_{n,m}.\end{equation*}

Finally, we have $H^{0}(\bar{X}_{m}, K_{\bar{X}_{m}})\simeq S_{d+m}({{\Gamma}}, \det)$ by [ 3 , theorem 3·1].

Remark 2·7. The case $k\geq d$ of Theorem 2·6 holds also when $d=1, 2$ . We put the assumption $d\geq 3$ for the requirement of the Koecher principle from [ 5 ]. Therefore, in fact, only the case $(d, k)=(2, 1)$ with Witt index 2 is not covered.