Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-25T01:33:23.639Z Has data issue: false hasContentIssue false
  • English
  • Français

Derangements and Laguerre polynomials

Published online by Cambridge University Press:  24 October 2008

S. Even  and
J. Gillis
S. Even
Affiliation:
Department of applied Mathematics, The Weizmann Institute of Science, Rehovot, Israel
J. Gillis
Affiliation:
Department of applied Mathematics, The Weizmann Institute of Science, Rehovot, Israel
Get access Rights & Permissions [Opens in a new window]

Abstract

Given a set consisting of n1, objects of type 1, n2 of type 2, …, nk of type k, we denote by the number of possible derangements of the set i.e. permutations in which no object occupies a site originally occupied by an object of the same type. A formula is found for 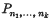in terms of Laguerre polynomials, and some of its implications are considered.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 79 , Issue 1 , January 1976 , pp. 135 - 143
Copyright
Copyright © Cambridge Philosophical Society 1976

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Erdélyi, A. et al. Higher transcendental functions, vol. 1 (New York; McGraw-Hill, 1953).Google Scholar
(2)Erdélyi, A. et al. Higher transcendental functions, vol. 2 (New York; McGraw-Hill, 1953).Google Scholar
(3)Gillis, J. and Weiss, G.Products of Laguerre Polynomials. Math. Comp. 14 (1960), 60.CrossRefGoogle Scholar
(4)Watson, G. N.A note on the polynomials of Hermite and Laguerre. J. London Math. Soc. 13 (1938), 29.CrossRefGoogle Scholar