No CrossRef data available.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
Published online by Cambridge University Press: 24 October 2008
The bisecant curves of a ruled surface, that is to say the curves on the surface which meet each generator in two points, are fundamental in the consideration of the normal space of the ruled surface. It is well known that if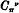 
is a bisecant curve of order ν and genus π on a ruled surface of order N and genus P, then
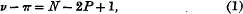
provided that the curve 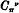has no double points which count twice as intersections of a generator of the ruled surface.
* Segre, , Math. Ann. 34 (1889), 2.CrossRefGoogle Scholar
† I hope to publish a paper on special ruled surfaces in the near future.
* Segre, , Math. Ann. 30 (1887), 222–223.Google Scholar
† In one of the papers referred to above Segre gives the impression that q is zero provided that the expression in brackets is not negative, but this is not true even with his supposition that n > 2p − 2; cf. Segre, , Math. Ann. 30 (1887), 222.Google Scholar
* The 2P points in which 
is met by C 1 and C 2 might offer less than 2P conditions, so that the number of further conditions to contain would be greater. But the conditions for a quadric to contain C 1 and C 2 would be less by the same amount, and the total number of conditions would be unaltered.
* Segre, , Math. Ann. 34 (1889), 7.CrossRefGoogle Scholar
* The only surface with two double lines is the R 41 in [3].
† If the curve is special the ruled surface given by the γ12 is a cone.
* See § 8.