Article contents
Artin's primitive root conjecture and a problem of Rohrlich
Published online by Cambridge University Press: 01 May 2014
Abstract
Let 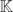 $\mathbb{K}$ be a number field, Γ a finitely generated subgroup of
$\mathbb{K}$ be a number field, Γ a finitely generated subgroup of 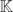 $\mathbb{K}$*, for instance the unit group of
$\mathbb{K}$*, for instance the unit group of 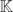 $\mathbb{K}$, and κ>0. For an ideal
$\mathbb{K}$, and κ>0. For an ideal  $\mathfrak{a}$ of
$\mathfrak{a}$ of 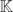 $\mathbb{K}$ let indΓ(
$\mathbb{K}$ let indΓ( $\mathfrak{a}$]></alt-text></inline-graphic>) denote the multiplicative index of the reduction of Γ in <inline-graphic name="S0305004114000206_inline3"><alt-text><![CDATA[$(\mathcal{O}_\mathbb{K}/\mathfrak{a})$* (whenever it makes sense). For a prime ideal
$\mathfrak{a}$]></alt-text></inline-graphic>) denote the multiplicative index of the reduction of Γ in <inline-graphic name="S0305004114000206_inline3"><alt-text><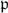 $\mathfrak{p}$ of
$\mathfrak{p}$ of 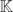 $\mathbb{K}$ and a positive integer γ let
$\mathbb{K}$ and a positive integer γ let 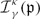 $\mathcal{I}_\gamma^\kappa(\mathfrak{p})$ be the average of
$\mathcal{I}_\gamma^\kappa(\mathfrak{p})$ be the average of 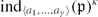 ${ind}_{\langle a_1,\dots,a_\gamma\rangle}(\mathfrak{p})^\kappa$ over all tupels
${ind}_{\langle a_1,\dots,a_\gamma\rangle}(\mathfrak{p})^\kappa$ over all tupels 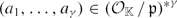 $(a_1,\dots,a_\gamma)\in{(\mathcal{O}_\mathbb{K}/\mathfrak{p})^*}^\gamma$. Motivated by a problem of Rohrlich we prove, partly conditionally on fairly standard hypotheses, lower bounds for
$(a_1,\dots,a_\gamma)\in{(\mathcal{O}_\mathbb{K}/\mathfrak{p})^*}^\gamma$. Motivated by a problem of Rohrlich we prove, partly conditionally on fairly standard hypotheses, lower bounds for 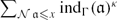 $\sum_{\mathcal{N}{\mathfrak{a}\leq x}{ind}_{\Gamma}({\mathfrak{a})^\kappa$ and asymptotic formulae for
$\sum_{\mathcal{N}{\mathfrak{a}\leq x}{ind}_{\Gamma}({\mathfrak{a})^\kappa$ and asymptotic formulae for 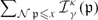 $\sum_{\mathcal{N}\mathfrak{p} \leq x} {\mathcal{I}_{\gamma}^\kappa({\mathfrak{p})$.
$\sum_{\mathcal{N}\mathfrak{p} \leq x} {\mathcal{I}_{\gamma}^\kappa({\mathfrak{p})$.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 157 , Issue 1 , July 2014 , pp. 79 - 99
- Copyright
- Copyright © Cambridge Philosophical Society 2014
References
REFERENCES
- 3
- Cited by


