Published online by Cambridge University Press: 24 October 2008
Let A denote the mod 2 Steenrod algebra. Let ℤ2[x, x−l] be the (graded) ring of finite Laurent series over ℤ2 in the variable x with dim (x) = 1. ℤ2[x, x−1] is a module over the Steenrod algebra A by
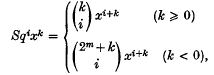
where 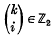 are binomial coefficients modulo 2 and m > 0 is large compared with |k| and i. Let M be the A-submodule of ℤ2[x, x−1 ] generated by all powers xi with i ≠ −1. It is easy to see that ℤ2 [,x, x−1]/M ≅ σ−1ℤ2 (means ℤ2 on dimension − 1). Let ρ: ℤ2[x, x−1] → σ−1 ℤ2 be the projection map.
are binomial coefficients modulo 2 and m > 0 is large compared with |k| and i. Let M be the A-submodule of ℤ2[x, x−1 ] generated by all powers xi with i ≠ −1. It is easy to see that ℤ2 [,x, x−1]/M ≅ σ−1ℤ2 (means ℤ2 on dimension − 1). Let ρ: ℤ2[x, x−1] → σ−1 ℤ2 be the projection map.