No CrossRef data available.
The action in a uniform field
Published online by Cambridge University Press: 24 October 2008
Extract
We consider the motion of a particle in a plane field of force. We take rectangular cartesian coordinates (x, y) in the plane, and denote by V(x, y) the potential of the field, and by h the (constant) energy of the motion. If A and B, whose coordinates are (x0, y0) and (x1, y1), are any two points on an orbit, the orbit is characterized by the property that
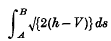
taken along the orbit is stationary as compared with the integral taken along a neighbouring curve joining the same points. Here s denotes the length of the are measured from A to B. This is one form, sometimes called Jacobi's form, of the principle of least action. The value of the integral, taken along the orbit, and expressed in terms of the coordinates of the termini and the constant of energy,
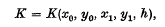
is the action function for the field V.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 37 , Issue 2 , April 1941 , pp. 168 - 176
- Copyright
- Copyright © Cambridge Philosophical Society 1941
References
* Bolza, O., Vorlesungen über Variationsrechnung (1933), p. 80.Google Scholar
* Bolza, ibid. p. 123.
* The formula for K was evaluated, by a different method from that given above, by Darboux, , Théorie Générale des Surfaces, 2 (1889), p. 448.Google Scholar
* Bolza, ibid. p. 398.
* Bolza, ibid. pp. 436–8.
† Todhunter, I., Researches in the calculus of variations (1871), p. 60.Google Scholar


