Hostname: page-component-cd9895bd7-gvvz8 Total loading time: 0 Render date: 2024-12-27T01:43:49.023Z Has data issue: false hasContentIssue false
  • English
  • Français

Remarks about inhomogeneous pair correlations

Part of: Diophantine approximation, transcendental number theory Probabilistic theory: distribution modulo $1$; metric theory of algorithms

Published online by Cambridge University Press:  06 September 2021

FELIPE A. RAMÍREZ
FELIPE A. RAMÍREZ*
Affiliation:
Department of Mathematics and Computer Science, Wesleyan University, Middletown, Connecticut, U.S.A e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Given an infinite subset 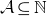$\mathcal{A} \subseteq\mathbb{N}$ , let A denote its smallest N elements. There is a rich and growing literature on the question of whether for typical 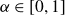$\alpha\in[0,1]$ , the pair correlations of the set 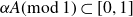$\alpha A (\textrm{mod}\ 1)\subset [0,1]$ are asymptotically Poissonian as N increases. We define an inhomogeneous generalisation of the concept of pair correlation, and we consider the corresponding doubly metric question. Many of the results from the usual setting carry over to this new setting. Moreover, the double metricity allows us to establish some new results whose singly metric analogues are missing from the literature.

MSC classification

Primary: 11J71: Distribution modulo one 11K38: Irregularities of distribution, discrepancy 11J83: Metric theory
Secondary: 11K60: Diophantine approximation 11K31: Special sequences
Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 173 , Issue 2 , September 2022 , pp. 369 - 386
Copyright
© The Author(s), 2021. Published by Cambridge University Press on behalf of Cambridge Philosophical Society

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

For Jorge A. Ramírez (1954–2020)—with Love and Gratitude

References

C., Aistleitner, T., Lachmann and F., Pausinger. Pair correlations and equidistribution. J. Number Theory 182 (2018), 206–220.Google Scholar
C., Aistleitner, T., Lachmann and N., Technau. There is no Khintchine threshold for metric pair correlations. Mathematika 65(4) (2019), 929–949.Google Scholar
C., Aistleitner, G., Larcher and M., Lewko. Additive energy and the Hausdorff dimension of the exceptional set in metric pair correlation problems. Israel J. Math. 222(1) (2017), 463–485. With an appendix by Jean Bourgain.Google Scholar
Bloom, T. F., S., Chow, A., Gafni and A., Walker. Additive energy and the metric Poissonian property. Mathematika 64(3) (2018), 679–700.10.1112/S0025579318000207CrossRefGoogle Scholar
Bloom, T. F. and A., Walker. GCD sums and sum-product estimates. Israel J. Math., 235(1) (2020), 1–11.10.1007/s11856-019-1932-0CrossRefGoogle Scholar
S., Grepstad and G., Larcher. On pair correlation and discrepancy. Arch. Math. (Basel) 109(2) (2017), 143–149.Google Scholar
A., Hinrichs, L., KaltenbÖck, G., Larcher, W., Stockinger and M., Ullrich. On a multi-dimensional Poissonian pair correlation concept and uniform distribution. Monatsh. Math. 190(2) (2019), 333–352.Google Scholar
T., Lachmann and N., Technau. On exceptional sets in the metric Poissonian pair correlations problem. Monatsh. Math. 189(1) (2019), 137–156.Google Scholar
G., Larcher and W., Stockinger. 7. On Pair Correlation of Sequences (De Gruyter, Berlin, Boston, 20 Jan. 2020), 133–146.Google Scholar
G., Larcher and W., Stockinger. Pair correlation of sequences with maximal additive energy. Math. Proc. Camb. Phil. Soc. 168(2) (2020), 287–293.10.1017/S030500411800066XCrossRefGoogle Scholar
G., Larcher and W., Stockinger. Some negative results related to Poissonian pair correlation problems. Discrete Math. 343(2) (2020), 111656, 11.Google Scholar
J., Marklof. Distribution modulo one and Ratner’s theorem. In Equidistribution in number theory, an introduction, volume 237 of NATO Sci. Ser. II Math. Phys. Chem. (Springer, Dordrecht, 2007), 217–244.10.1007/978-1-4020-5404-4_11CrossRefGoogle Scholar
J., Marklof. Pair correlation and equidistribution on manifolds. Monatshefte für Mathematik, 191 (2020) 279–294.Google Scholar
Z., Rudnick and P., Sarnak. The pair correlation function of fractional parts of polynomials. Comm. Math. Phys. 194(1) (1998), 61–70.Google Scholar
T., Tao and V., Vu. Additive combinatorics. Camb. Stud. Adv. Math. (Cambridge University Press, Cambridge, 2006).10.1017/CBO9780511755149CrossRefGoogle Scholar
A., Walker. The primes are not metric Poissonian. Mathematika 64(1) (2018), 230–236.Google Scholar
A., Walker. Additive combinatorics: some new techniques for pair correlation problems. Notes for a minicourse (2019).Google Scholar