Rate of growth and convergence factors for power methods of limitation

Extract

Let 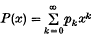, where p_k are complex numbers, have 0 < ρ ≤ ∞ for radius of convergence and assume that P(x) ≠ 0 for α ≤ x < ρ (α < ρ is some real constant). Assuming that 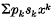is convergent for all (x ∈ [0, ρ), we define the P-limit of the sequence s = {s_k} by

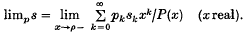
This, so called, power method of limitation (see (3), Definition 9 and (1) Definition 6) will be denoted by P. The best known power methods are Abel's (P(x) = 1/(1 – x), α = 0, ρ = 1) and Borel's (P(x) = e^x, α = 0, ρ = ∞). By C_p we denote the set of all sequences, P-limitable to a finite limit and by 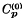the set of all sequences, P-limitable to zero.