Article contents
Projections of Poisson cut-outs in the Heisenberg group and the visual 3-sphere
Published online by Cambridge University Press: 08 March 2021
Abstract
We study projectional properties of Poisson cut-out sets E in non-Euclidean spaces. In the first Heisenbeg group 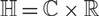 \[\mathbb{H} = \mathbb{C} \times \mathbb{R}\], endowed with the Korányi metric, we show that the Hausdorff dimension of the vertical projection
\[\mathbb{H} = \mathbb{C} \times \mathbb{R}\], endowed with the Korányi metric, we show that the Hausdorff dimension of the vertical projection 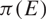 \[\pi (E)\] (projection along the center of
\[\pi (E)\] (projection along the center of  \[\mathbb{H}\]) almost surely equals
\[\mathbb{H}\]) almost surely equals 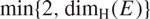 \[\min \{ 2,{\dim _\operatorname{H} }(E)\} \] and that
\[\min \{ 2,{\dim _\operatorname{H} }(E)\} \] and that 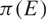 \[\pi (E)\] has non-empty interior if
\[\pi (E)\] has non-empty interior if 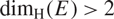 \[{\dim _{\text{H}}}(E) > 2\]. As a corollary, this allows us to determine the Hausdorff dimension of E with respect to the Euclidean metric in terms of its Heisenberg Hausdorff dimension
\[{\dim _{\text{H}}}(E) > 2\]. As a corollary, this allows us to determine the Hausdorff dimension of E with respect to the Euclidean metric in terms of its Heisenberg Hausdorff dimension 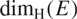 \[{\dim _{\text{H}}}(E)\].
\[{\dim _{\text{H}}}(E)\].
We also study projections in the one-point compactification of the Heisenberg group, that is, the 3-sphere 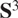 \[{{\text{S}}^3}\] endowed with the visual metric d obtained by identifying
\[{{\text{S}}^3}\] endowed with the visual metric d obtained by identifying 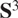 \[{{\text{S}}^3}\] with the boundary of the complex hyperbolic plane. In
\[{{\text{S}}^3}\] with the boundary of the complex hyperbolic plane. In 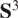 \[{{\text{S}}^3}\], we prove a projection result that holds simultaneously for all radial projections (projections along so called “chains”). This shows that the Poisson cut-outs in
\[{{\text{S}}^3}\], we prove a projection result that holds simultaneously for all radial projections (projections along so called “chains”). This shows that the Poisson cut-outs in  \[{{\text{S}}^3}\] satisfy a strong version of the Marstrand’s projection theorem, without any exceptional directions.
\[{{\text{S}}^3}\] satisfy a strong version of the Marstrand’s projection theorem, without any exceptional directions.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 172 , Issue 1 , January 2022 , pp. 197 - 230
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press on behalf of Cambridge Philosophical Society
Footnotes
Supported by: Academy of Finland via the Centre of Excellence in Analysis and Dynamics research and the research project Geometry of subRiemannian Groups (#288501), the European Research Council via the ERC Starting Grant #713998 GeoMeG ‘Geometry of Metric Groups’, the Institute Mittag-Leffler via the Fractal Geometry and Dynamics research program.
References
- 1
- Cited by


