Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-25T13:18:48.041Z Has data issue: false hasContentIssue false
  • English
  • Français

Paranormed sequence spaces generated by infinite matrices

Published online by Cambridge University Press:  24 October 2008

I. J. Maddox
I. J. Maddox
Affiliation:
University of Lancaster
Get access Rights & Permissions [Opens in a new window]

Abstract

A paranormed space X = (X, g) is a topological linear space in which the topology is given by paranorm g—a real subadditive function on X such that g(θ) = 0, g(x) = g(−x) and such that multiplication is continuous. In the above, θ is the zero in the complex linear space X and continuity of multiplication means that λn → λ, xn → x(i.e. g(xn − x) → 0) imply λnxn → λx, for scalars λ and vectors x. We shall use the term semimetric function to describe a real subadditive function g on X such that g(θ) = 0, g(x) = g(−x). Two familiar paranormed sequence spaces, which have been extensively studied (3), are l(p) and m(p). 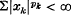For a given sequence p = (gk) of strictly positive numbers, l;(p) is the set of all complex sequences x = (xk) such that and m(p) is the set of x such that sup 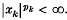Throughout, sums and suprema without limits are taken from 1 to ∞. Simons (3) considered only the case in which 0 < pk ≤ 1 so that natural paranorms would seem to be 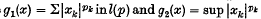in m(p). In fact Simons showed that g1 was a paranorm for l(p), but that g2 did not satisfy the continuity of multiplication axiom.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 64 , Issue 2 , April 1968 , pp. 335 - 340
Copyright
Copyright © Cambridge Philosophical Society 1968

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Maddox, I J.On Kuttner's theorem. J. London Math. Soc. (to appear shortly).Google Scholar
(2)Maddox, I. J.Spaces of strongly sun-unable sequences. Quarterly J. Math. (Oxford) (to appear shortly).Google Scholar
(3)Simons, S.The sequence spaces l(pv) and m(pv). Proc. London Math. Soc. (3), 15 (1965), 422–36.CrossRefGoogle Scholar