Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
James, I.M.
1978.
On category, in the sense of Lusternik-Schnirelmann.
Topology,
Vol. 17,
Issue. 4,
p.
331.
Singhof, Wilhelm
1979.
Minimal coverings of manifolds with balls.
Manuscripta Mathematica,
Vol. 29,
Issue. 2-4,
p.
385.
Byrnes, Christopher I.
1980.
Geometrical Methods for the Theory of Linear Systems.
p.
85.
Hiller, Howard L.
1980.
On the cohomology of real Grassmanians.
Transactions of the American Mathematical Society,
Vol. 257,
Issue. 2,
p.
521.
Brockett, R.
and
Byrnes, C.
1981.
Multivariable Nyquist criteria, root loci, and pole placement: A geometric viewpoint.
IEEE Transactions on Automatic Control,
Vol. 26,
Issue. 1,
p.
271.
Byrnes, Christopher I.
1982.
Analysis and Optimization of Systems.
Vol. 44,
Issue. ,
p.
20.
Byrnes, Christopher I.
and
Stevens, Peter K.
1982.
Feedback Control of Linear and Nonlinear Systems.
Vol. 39,
Issue. ,
p.
9.
Stong, R.E.
1982.
Cup products in Grassmannians.
Topology and its Applications,
Vol. 13,
Issue. 1,
p.
103.
Byrnes, Christopher I.
and
Duncan, Tyrone E.
1982.
New Directions in Applied Mathematics.
p.
29.
1982.
Vector Bundles.
Vol. 101,
Issue. ,
p.
303.
Ziller, Wolfgang
1983.
Geometry of the Katok examples.
Ergodic Theory and Dynamical Systems,
Vol. 3,
Issue. 1,
p.
135.
Karcanias, N.
and
Giannakopoulos, C.
1989.
Necessary and sufficient conditions for zero assignment by constant squaring down.
Linear Algebra and its Applications,
Vol. 122-124,
Issue. ,
p.
415.
Byrnes, C. I.
1989.
Three Decades of Mathematical System Theory.
Vol. 135,
Issue. ,
p.
31.
Jaworowski, Jan
1991.
Algebraic Topology Poznań 1989.
Vol. 1474,
Issue. ,
p.
231.
Leventides, J.
and
Karcanias, N.
1992.
Pole placement via PI controllers: necessary and sufficient conditions.
p.
161.
Wang, Xiaochang
1994.
Decentralized Pole Assignment and Product Grassmannians.
SIAM Journal on Control and Optimization,
Vol. 32,
Issue. 3,
p.
855.
Ravi, M.S.
Rosenthal, J.
and
Wang, X.A.
1995.
Dynamic pole assignment for systems in generalized first order form: a report on results derived by algebro-geometric techniques.
Vol. 2,
Issue. ,
p.
1900.
Ravi, M. S.
Rosenthal, Joachim
and
Wang, Xiaochang
1996.
Dynamic Pole Assignment and Schubert Calculus.
SIAM Journal on Control and Optimization,
Vol. 34,
Issue. 3,
p.
813.
Pintea, Cornel
1998.
A measure of non-immersability of the Grassmann manifolds in some euclidean spaces.
Proceedings of the Edinburgh Mathematical Society,
Vol. 41,
Issue. 1,
p.
197.
Byrnes, Christopher I.
1999.
Mathematical Control Theory.
p.
99.


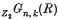 is strictly less than the maximum possible value dim
is strictly less than the maximum possible value dim 