Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-27T02:46:22.807Z Has data issue: false hasContentIssue false
  • English
  • Français

On the dimension of the non-Cohen–Macaulay locus of local rings admitting dualizing complexes

Published online by Cambridge University Press:  24 October 2008

Nguyen Tu Cuong
Nguyen Tu Cuong
Affiliation:
Institute of Mathematics, P.O. Box 631 Bo Ho, 10.000 Hanoi, Vietnam
Get access Rights & Permissions [Opens in a new window]

Extract

In this paper we mainly consider local rings admitting dualizing complexes. It is well-known that if a Noetherian local ring A admits a dualizing complex, then the non-Cohen–Macaulay (abbreviated CM) locus of A is closed in the Zariski topology (cf. [8, 10]). If the dimension of this locus is zero and A is equidimensional, i.e. the punctured spectrum of A is locally CM and dim(A/P) = dim (A) for all minimal prime ideals P ∈ Ass (A), then A is a generalized CM ring and its structure is well-understood (see [2, 12]). For instance, one of the characterizations of generalized CM rings is the conditions that for any parameter ideal q contained in a large power of the maximal ideal m of A, the difference between length and multiplicity

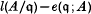
is independent of the choice of q. However, if the dimension of the non-CM locus is larger than zero, little is known about how this dimension is related to the structure of the local ring A. The purpose of this paper is to show that if M is a finitely generated A-module, then there exist systems of parameters x = (x1, …, xd) (where d = dim M) such that the difference

is a polynomial in n1, …, nd for all positive integers n1, …, nd and the degree of IM(n1, …, nd;x) is independent of the choice of x. We shall also give various characterizations of this degree by using the notion of reducing systems of parameters of Auslander and Buchsbaum[l]. In particular, if the module M is equidimensional we shall show that the degree of IM(n1, …, nd;x) is equal to the dimension of the non-CM locus of M.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 109 , Issue 3 , May 1991 , pp. 479 - 488
Copyright
Copyright © Cambridge Philosophical Society 1991

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Auslander, M. and Buchsbaum, D. A.. Codimension and multiplicity. Ann. of Math. 68 (1958), 625–657.CrossRefGoogle Scholar
[2]Cuong, N. T., Schenzel, P. and Trung, N. V.. Verallgemeinerte Cohen–Macaulay Moduln. Math. Nachr. 85 (1978), 57–73.Google Scholar
[3]Cuong, N. T.. On the length of the powers of systems of parameters in local rings. Nagoya Math. J. 120 (1990).CrossRefGoogle Scholar
[4]Garcia Roig, J.-L.. On polynomial bounds for the Koszul homology of certain multiplicity systems. J. London Math. Soc. (2) 34 (1986), 411–416.CrossRefGoogle Scholar
[5]Grothendieck, A.. SGA II (North-Holland, 1962).Google Scholar
[6]Hartshorne, R.. Algebraic Geometry (Springer-Verlag, 1977).CrossRefGoogle Scholar
[7]Matsumura, H.. Commtrtatrve Algebra (Benjamin, New York, 1980).Google Scholar
[8]Schenzel, P.. Einige Anwendungen der lokalen Dualität und verallgemeinerte Cohen-Macaulay Moduln. Math. Nachr. 69 (1975), 227–242.CrossRefGoogle Scholar
[9]Schenzel, P.. Dualisierende Komplexe in der lokalen Algebra und Buchsbaum Ringe. Lecture Notes in Math. vol. 907 (Springer-Verlag, 1982).CrossRefGoogle Scholar
[10]Sharp, R. Y.. A commutative Noetherian ring which possesses a dualizing complex is acceptable. Math. Proc. Cambridge Philos. Soc. 82 (1977), 197–213.CrossRefGoogle Scholar
[11]Sharp, R. Y. and Hamieh, M. A.. Lengths of certain generalized fractions. J. Pure Appl. Algebra 38 (1985), 323–336.CrossRefGoogle Scholar
[12]Trung, N. V.. Toward a theory of generalized Cohen–Macaulay modules. Nagoya Math. J. 102 (1986), 1–49.CrossRefGoogle Scholar