Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-25T13:49:11.024Z Has data issue: false hasContentIssue false
  • English
  • Français

On critical pairs of product sets in a certain matrix group

Published online by Cambridge University Press:  24 October 2008

M. McCrudden
M. McCrudden
Affiliation:
University of Birmingham
Get access Rights & Permissions [Opens in a new window]

Extract

1. Introduction. If G is a locally compact Hausdorff topological group, and μ is (left) Haar measure on G, then we denote by ℬ(G) the class of all Borel subsets of G having finite measure, and by VG the set {μ(E): E ∊ ℬ(G)} of real numbers. The product set function of G, ΦG: VG × VG → VG, is defined (see (4) and (5)) by

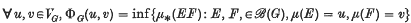
and, for each u, v ∈ VG, we call a pair (E, F) of Borel subsets of G a critical (u, v)-pair, if μ(E) = u, μ(F) = v, and μ*(EF) = ΦG(u, v). We denote the class of all critical (u, v)-pairs by and we write ℰG for .

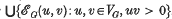Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 67 , Issue 3 , May 1970 , pp. 569 - 581
Copyright
Copyright © Cambridge Philosophical Society 1970

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Halmos, P.Measure Theory. (New York, 1959).Google Scholar
(2)Henstock, R. and Macbeath, A. M.On the measure of sum-sets, 1. Proc. London Math. Soc. 3 (1953), 182–194.Google Scholar
(3)Kneser, M.Summenmengen in lokalkompakten Abelschen Gruppen. Math. Z. 66 (1956), 88–110.Google Scholar
(4)Macbeath, A. M.On the measure of product sets in a topological group. J. London Math. Soc. 35 (1960), 403–407.Google Scholar
(5)McCrudden, M.On product sets of a unimodular group. Proc. Cambridge Philos. Soc. 64 (1968), 1001–1007.Google Scholar
(6)McCrudden, M.On the Brunn-Minkowski coefficient of a locally compact unimodular group. Proc. Cambridge Philos. Soc. 65 (1969), 33–45.Google Scholar