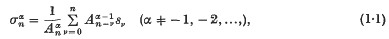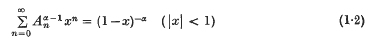Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Kangro, G. F.
1976.
Theory of summability of sequences and series.
Journal of Soviet Mathematics,
Vol. 5,
Issue. 1,
p.
1.
Kanno, Kôsi
1978.
On the absolute Nörlund summability factors of a Fourier series and its conjugate series at a point.
Bulletin of the Australian Mathematical Society,
Vol. 19,
Issue. 2,
p.
161.


 .
.