On a Sturm-Liouville expansion in series of Bessel functions
Published online by Cambridge University Press: 24 October 2008
Extract
1. The determination of potentials and wave functions defined in regions bounded by the natural coordinate surfaces of a cylindrical polar coordinate system leads to eigenvalue problems connected with Bessel's differential equation
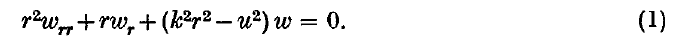
We shall consider the annulus 0 < a ≤ r ≤ b and take first the boundary condition to be that of vanishing on both surfaces. It is then convenient to introduce the function

This function will automatically satisfy the condition of vanishing for r = a. It will also vanish on r = b if u and k are related by the condition that ψ(u, k, b) = 0. If we regard u as being fixed and real then there will be an infinite number of zeros kn(u)(n = 1,2,…).
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 62 , Issue 1 , January 1966 , pp. 61 - 72
- Copyright
- Copyright © Cambridge Philosophical Society 1966
References
REFERENCES
- 5
- Cited by


