Hostname: page-component-cd9895bd7-hc48f Total loading time: 0 Render date: 2024-12-25T13:41:55.192Z Has data issue: false hasContentIssue false
  • English
  • Français

On a conjecture of Carter concerning irreducible Specht modules

Published online by Cambridge University Press:  24 October 2008

G. D. James
G. D. James
Affiliation:
Department of Pure Mathematics and Mathematical Statistics, Cambridge
Get access Rights & Permissions [Opens in a new window]

Extract

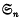We study the question: Which ordinary irreducible representations of the symmetric group remain irreducible modulo a prime p?

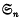Let Sλ be the Specht module corresponding to the partition λ of n. The definition of Sλ is ‘independent of the field we are working over’. When the field has characteristic zero, Sλ is irreducible, and gives the ordinary irreducible representation of corresponding to the partition λ. Thus we are interested in the problem of whether or not Sλ is irreducible over a field of characteristic p.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 83 , Issue 1 , January 1978 , pp. 11 - 17
Copyright
Copyright © Cambridge Philosophical Society 1978

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1) James, G. D. Representations of the symmetric groups over the field of order 2. J. Algebra 38 (1976), 280–308.CrossRefGoogle Scholar
(2) James, G. D. The irreducible representations of the symmetric groups, Bull. London Math. Soc. 8 (1976), 229–232.CrossRefGoogle Scholar
(3) James, G. D. On the decomposition matrices of the symmetric groups: I. J. Algebra 43 (1976), 42–44.CrossRefGoogle Scholar
(4) James, G. D. A characteristic-free approach to the representation theory of . J. Algebra 46 (1977), 430–450.CrossRefGoogle Scholar
(5) James, G. D. Some combinatorial results involving Young diagrams. Math. Proc. Cambridge Philon. Soc. 83 (1978), 1–10.CrossRefGoogle Scholar
(6) Kerber, A. Representations of permutation groups, Lecture Notee in Mathematics, no. 240.Google Scholar
(7) Payne, M. T. J. Ph.D. Thesis, University of Warwick.Google Scholar