Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-25T00:56:42.447Z Has data issue: false hasContentIssue false
  • English
  • Français

Note on propagation of classical waves: II

Published online by Cambridge University Press:  24 October 2008

H. Kanseta
H. Kanseta
Affiliation:
Tokushima University, Japan
Get access Rights & Permissions [Opens in a new window]

Extract

1. Main results and notations. We consider Cauchy's problem for the classical wave equation in :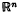

with initial conditions u(0, x) = uo(x) and ∂tu(0, x) = u1 (x). In (1·1) δ and m2 stand for the Laplacian in 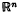and a constant respectively. Note that a second order hyper-bolic differential equation with real constants can be reduced to (1·1) ([1], p. 183). Let uj (j = 0,1) be C∞ funotions on 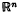whose support is contained in the closed ball BR = {xεl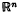; |x| ≥R} for some R > 0. In this note we shall show that the solution u(t, x) of (1·1) possesses the following properties.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 98 , Issue 2 , September 1985 , pp. 367 - 372
Copyright
Copyright © Cambridge Philosophical Society 1985

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Courant, R. and Hilbert, D.Methods of Mathematical Physics, vol. 2 (Interscience Publishers, 1962).Google Scholar
[2]Freedlakdeb, F. G.The Wave Equation in a Curved Space-time (Cambridge University-Press, 1975).Google Scholar
[3]Gel'fand, I. M. and Shilov, G. E.Generalized Functions, vol. 1 (Academic Press, 1964).Google Scholar
[4]Kaneta, H.Note on propagation of classical waves: I. Math. Proc. Cambridge Philos. Soc. 98 (1985), 179–181.CrossRefGoogle Scholar
[5]Mizohata, S.The Theory of Partial Differential Equations (Cambridge University Press, 1973).Google Scholar
[6]Watson, G. N.A treatise on the theory of Besselfunctions (Cambridge University Press, 1922).Google Scholar