Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-25T13:41:52.186Z Has data issue: false hasContentIssue false
  • English
  • Français

Locally compact groups with closed subgroups open and p-adic

Published online by Cambridge University Press:  24 October 2008

Karl H. Hofmann ,
Sidney A. Morris ,
Sheila Oates-Williams  and
V. N. Obraztsov
Karl H. Hofmann
Affiliation:
Fachbereich Mathematik, Technische Hochschule Darmstadt, Schlonssgartenstrasse 7, D-6100 Darmstadt, Germany e-mail: [email protected]
Sidney A. Morris
Affiliation:
Faculty of Informatics, The University of Wollongong, Wollongong, NSW 2522, Australia, e-mail: [email protected]
Sheila Oates-Williams
Affiliation:
Department of Mathematics, The University of Queensland, Qld 4072, Australia e-mail: [email protected]
V. N. Obraztsov
Affiliation:
Department of Mathematics and Physics, Kostroma Teachers' Training Institute, Kostroma 156601, Russia
Get access Rights & Permissions [Opens in a new window]

Extract

An open subgroup U of a topological group G is always closed, since U is the complement of the open set 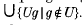. An arbitrary closed subgroup C of G is almost never open, unless G belongs to a small family of exceptional groups. In fact, if G is a locally compact abelian group in which every non-trivial subgroup is open, then G is the additive group δp of p-adic integers or the additive group Ωp of p-adic rationale (cf. Robertson and Schreiber[5[, proposition 7). The fact that δp has interesting properties as a topological group has many roots. One is that its character group is the Prüfer group ℤp∞, which makes it unique inside the category of compact abelian groups. But even within the bigger class of not necessarily abelian compact groups the p-adic group δp is distinguished: it is the only one all of whose non-trivial subgroups are isomorphic (cf. Morris and Oates-Williams[2[), and it is also the only one all of whose non-trivial closed subgroups have finite index (cf. Morris, Oates-Williams and Thompson [3[).

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 118 , Issue 2 , September 1995 , pp. 303 - 313
Copyright
Copyright © Cambridge Philosophical Society 1995

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1[Ivanov, S. V. and Ol'shanskiî, A. Yu.. Some applications of graded diagrams in combinatorial group theory. Groups, St Andrews 1989, London Math. Soc. Lecture Note Ser. 160 (1991), 258–308.Google Scholar
[2[Morris, Sidney A. and Oates-Williams, Sheila. A characterization of the topological group of p–adic integers. Butt. London Math. Soc. 19 (1987), 57–59.CrossRefGoogle Scholar
[3[Morris, Sidney A., Oates-Williams, Sheila and Thompson, H. B.. Locally compact groups with every closed subgroup of finite index. Bull. London Math. Soc. 22 (1990), 359–361.CrossRefGoogle Scholar
[4[Ol'shanskiî, A. Yu.. Geometry of Defining Relations. Mathematics and its applications (Soviet Series), vol. 70 (Kluwer Academic Publishers, 1991).Google Scholar
[5[Robertson, I. and Schreiber, B.. The additive structure of integer groups and p–adic number fields. Proc. Amer. Math. Soc. 19 (1968), 1453–1456.Google Scholar