Published online by Cambridge University Press: 24 October 2008
V. P. Snaith has shown that a number of interesting homology theories (e.g. K-theory, complex cobordism) arise when one localizes the stable homotopy type of certain H-spaces (BU(1), BU, etc.). In this paper we sketch a construction which, given an H-space X and 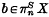 , produces a ring spectrum IΣ−nX which lies between Xand its localization with respect to b, and in many cases has a tractable structure. We apply this when X is an Eilenberg-MacLane space K(ℤ/, n) and
, produces a ring spectrum IΣ−nX which lies between Xand its localization with respect to b, and in many cases has a tractable structure. We apply this when X is an Eilenberg-MacLane space K(ℤ/, n) and 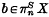 is a generator (p = 0 or a prime), and get complete results on the localizations in this case. The results confirm one's suspicions that localization is a fairly drastic manoeuvre, cutting down the large and complicated ring
is a generator (p = 0 or a prime), and get complete results on the localizations in this case. The results confirm one's suspicions that localization is a fairly drastic manoeuvre, cutting down the large and complicated ring  to something more or less trivial, except when p = 0 and n = 2, in which case the rich geometry of K(ℤ, 2) = BU(1) gives complex K-theory.
to something more or less trivial, except when p = 0 and n = 2, in which case the rich geometry of K(ℤ, 2) = BU(1) gives complex K-theory.