K-theory of real algebraic surfaces and threefolds
Published online by Cambridge University Press: 24 October 2008
Extract
Let X be an affine real algebraic variety, i.e., up to biregular isomorphism an algebraic subset of ℝn. (For definitions and notions of real algebraic geometry we refer the reader to the book [6].) Let  denote the ring of regular functions on X ([6], chapter 3). (If X is an algebraic subset of ℝn then
denote the ring of regular functions on X ([6], chapter 3). (If X is an algebraic subset of ℝn then  is comprised of all functions of the form f/g, where g, f: X → ℝ are polynomial functions with g−1(O) = Ø.) In this paper, assuming that X is compact, non-singular, and that dim X ≤ 3, we compute the Grothendieck group
is comprised of all functions of the form f/g, where g, f: X → ℝ are polynomial functions with g−1(O) = Ø.) In this paper, assuming that X is compact, non-singular, and that dim X ≤ 3, we compute the Grothendieck group 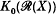 of projective modules over
of projective modules over  (cf. Section 1), and the Grothendieck group
(cf. Section 1), and the Grothendieck group  and the Witt group
and the Witt group  of symplectic spaces over
of symplectic spaces over  (cf. Section 2), in terms of the algebraic cohomology groups
(cf. Section 2), in terms of the algebraic cohomology groups  and
and  generated by the cohomology classes associated with the algebraic subvarieties of X. We also relate the group
generated by the cohomology classes associated with the algebraic subvarieties of X. We also relate the group 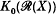 to the Grothendieck group KO(X) of continuous real vector bundles over X, and the groups
to the Grothendieck group KO(X) of continuous real vector bundles over X, and the groups  and
and  to the Grothendieck group K(X) of continuous complex vector bundles over X.
to the Grothendieck group K(X) of continuous complex vector bundles over X.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 106 , Issue 3 , November 1989 , pp. 471 - 480
- Copyright
- Copyright © Cambridge Philosophical Society 1989
References
REFERENCES
- 9
- Cited by


