Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-25T01:29:41.548Z Has data issue: false hasContentIssue false
  • English
  • Français

Invariant functions on matrices

Published online by Cambridge University Press:  24 October 2008

Stephen Donkin
Stephen Donkin
Affiliation:
School of Mathematical Sciences, Queen Mary and Westfield College, Mile End Road, London El 4NS
Get access Rights & Permissions [Opens in a new window]

Extract

The ring of symmetric polynomials in n variables may be interpreted as a ring of characters of the general linear group GL(n) (see e.g. [4], §3·5). 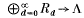We consider here a generalization of symmetric function theory in which we deal with trace functions on several matrices over ℂ An important feature of the ‘single matrix variable’ (or classical) case is the characteristic isomorphism (see [5], chapter I, §7) ch: R = between the graded ring R, whose component Rd in degree d is the character group of the symmetric group of degree d, and the ring of symmetric functions Λ in infinitely many variables (obtained from symmetric functions in n variables by taking the graded inverse limit with respect to n).

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 113 , Issue 1 , January 1993 , pp. 23 - 43
Copyright
Copyright © Cambridge Philosophical Society 1993

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Donkin, S.. On Schur algebras and related algebras II. J. Algebra 111 (1987), 354–364.Google Scholar
[2]Donkin, S.. Invariants of several matrices. Invent. Math., to appear.Google Scholar
[3]Green, J. A.. The characters of the finite general linear groups. Trans. Amer. Math. Soc. 80 (1955), 402–447.CrossRefGoogle Scholar
[4]Green, J. A.. Polynomial Representations of GLn. Lecture Notes in Math. vol. 830 (Springer-Verlag, 1980).Google Scholar
[5]Macdonald, I. G.. Symmetric Functions and Hall Polynomials (Oxford University Press, 1979).Google Scholar
[6]Procesi, C.. The invariant theory of n × n-matrices. Adv. in Math. 19 (1976), 306–381.CrossRefGoogle Scholar
[7]Sibirski, K. S.. On unitary and orthogonal matrix invariants. Dokl. Akad. Nauk. SSSR 172 (1967), 40–43.Google Scholar