Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-26T01:05:20.231Z Has data issue: false hasContentIssue false
  • English
  • Français

Harmonic analysis of scalar and vector fields in n

Published online by Cambridge University Press:  24 October 2008

J. Denmead Smith
J. Denmead Smith
Affiliation:
Department of Mathematics, University of Reading
Get access Rights & Permissions [Opens in a new window]

Abstract

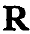It is shown that a real scalar function in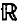 n which is of class Cn and either has zero mean on all spheres of unit radius, or has zero mean in all balls of unit radius admits a unique expansion in terms of eigenfunctions of the Laplacian operator. In a similar manner, a suitably smooth vector-valued function in 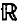n which has zero flux through all spheres cf unit radius is shown to be decomposable as the sum of a solenoidal part and a series of conservative parts that are eigenfunctions of the Laplacian. Applications are given, including some in complex analysis.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 72 , Issue 3 , November 1972 , pp. 403 - 416
Copyright
Copyright © Cambridge Philosophical Society 1972

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Bochner, S.Lectures on Fourier integrals (Princeton, 1959).CrossRefGoogle Scholar
(2)Courant, R. and Hilbert, D.Methods of mathematical physics, volume II (Interscience, New York, 1962).Google Scholar
(3)John, F.Bestimmung einer Funktion aus ihren Integralen über gewisse Mannigfaltigkeiten. Math. Ann. 109 (1933–1934), 488–520.CrossRefGoogle Scholar
(4)John, F.Abhängigkeiten zwischen den Flächenintegralen einer stetigen Funktion. Math. Ann. 111 (1935), 541–559.CrossRefGoogle Scholar
(5)John, F.Plane waves and spherical means applied to partial differential equations (Interscience, New York, 1955).Google Scholar
(6)Watson, G. N.Theory of Bessel functions, 2nd Edition (Cambridge, 1944).Google Scholar