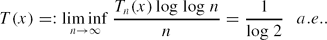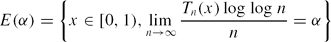Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Wu, Jun
and
Xu, Jian
2011.
On the distribution for sums of partial quotients in continued fraction expansions.
Nonlinearity,
Vol. 24,
Issue. 4,
p.
1177.
Zhang, Zhen-Liang
2012.
On sets of exact Diophantine approximation over the field of formal series.
Journal of Mathematical Analysis and Applications,
Vol. 386,
Issue. 1,
p.
378.
SHEN, LUMING
XU, JIAN
and
JING, HUIPING
2013.
ON THE LARGEST DEGREE OF THE PARTIAL QUOTIENTS IN CONTINUED FRACTION EXPANSIONS OVER THE FIELD OF FORMAL LAURENT SERIES.
International Journal of Number Theory,
Vol. 09,
Issue. 05,
p.
1237.
Zhang, Zhenliang
and
Lü, Meiying
2016.
The relative growth rate of the largest partial quotient to the sum of partial quotients in continued fraction expansions.
Journal of Number Theory,
Vol. 163,
Issue. ,
p.
482.
LIAO, LINGMIN
and
RAMS, MICHAŁ
2016.
Subexponentially increasing sums of partial quotients in continued fraction expansions.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 160,
Issue. 3,
p.
401.
WANG, BAO-WEI
WU, JUN
and
XU, JIAN
2016.
A generalization of the Jarník–Besicovitch theorem by continued fractions.
Ergodic Theory and Dynamical Systems,
Vol. 36,
Issue. 4,
p.
1278.
Zhang, Zhenliang
2016.
The relative growth rate of the largest digit in continued fraction expansions.
Lithuanian Mathematical Journal,
Vol. 56,
Issue. 1,
p.
133.
Ma, Liangang
2017.
A remark on Liao and Rams' result on the distribution of the leading partial quotient with growing speed en1/2 in continued fractions.
Comptes Rendus. Mathématique,
Vol. 355,
Issue. 7,
p.
734.
Song, Kunkun
Fang, Lulu
and
Ma, Jihua
2017.
Level sets of partial maximal digits for Lüroth expansion.
International Journal of Number Theory,
Vol. 13,
Issue. 10,
p.
2777.
Liu, Weibin
and
Li, Bing
2017.
Chaotic and topological properties of continued fractions.
Journal of Number Theory,
Vol. 174,
Issue. ,
p.
369.
CHANG, JINHUA
and
CHEN, HAIBO
2018.
Slow increasing functions and the largest partial quotients in continued fraction expansions.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 164,
Issue. 1,
p.
1.
Ma, Liangang
2018.
Full Dimensional Sets of Reals Whose Sums of Partial Quotients Increase in Certain Speed.
Journal of Number Theory,
Vol. 190,
Issue. ,
p.
254.
FANG, LULU
and
LIU, JIAN
2021.
ON THE LARGEST PARTIAL QUOTIENTS IN CONTINUED FRACTION EXPANSIONS.
Fractals,
Vol. 29,
Issue. 04,
p.
2150099.
Fang, Lulu
Ma, Jihua
and
Song, Kunkun
2021.
Some exceptional sets of Borel–Bernstein theorem in continued fractions.
The Ramanujan Journal,
Vol. 56,
Issue. 3,
p.
891.
Zhang, Mengjie
and
Ma, Chao
2021.
On the exceptional sets concerning the leading partial quotient in continued fractions.
Journal of Mathematical Analysis and Applications,
Vol. 500,
Issue. 1,
p.
125110.
SHANG, LEI
and
WU, MIN
2022.
SOME RESULTS ON THE LARGEST PARTIAL QUOTIENT IN CONTINUED FRACTIONS.
Fractals,
Vol. 30,
Issue. 04,
LÜ, MEIYING
and
ZHANG, ZHENLIANG
2022.
ON THE INCREASING PARTIAL QUOTIENTS OF CONTINUED FRACTIONS OF POINTS IN THE PLANE.
Bulletin of the Australian Mathematical Society,
Vol. 105,
Issue. 3,
p.
404.
Lin, Shuyi
Li, Jinjun
and
Lou, Manli
2022.
Exceptional sets related to the largest digits in Lüroth expansions.
International Journal of Number Theory,
Vol. 18,
Issue. 07,
p.
1429.
WANG, JINFENG
and
ZHANG, YUAN
2022.
METRIC THEORY OF PARTIAL QUOTIENTS OF N-CONTINUED FRACTIONS.
Fractals,
Vol. 30,
Issue. 01,
ZHANG, MENGJIE
and
WANG, WEILIANG
2023.
ON LÜROTH EXPANSIONS IN WHICH THE LARGEST DIGIT GROWS WITH SLOWLY INCREASING SPEED.
Bulletin of the Australian Mathematical Society,
Vol. 107,
Issue. 2,
p.
204.