Compact operators and Banach algebras

Extract

1. Introduction. Let X be a complex Banach space and let 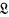(X) denote the Banach algebra of all bounded linear operators on X. In this paper we study subalgebras 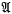of 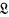(X) that contain non-zero compact operators and that are Banach algebras with respect to some norm dominating the operator norm. Our main aim is to give conditions for the existence of minimal one-sided ideals in 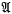. Barnes ((l) Theorem 2·2) has shown that if every element of a semi-simple Banach algebra has countable spectrum then the algebra has minimal one-sided ideals. If, in particular, 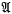is a uniformly closed sub-algebra of the compact operators on X, then every element of 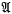has countable spectrum by the Riesz–Schauder theory and so 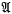has minimal one-sided ideals. We extend this latter result by showing that if 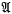is a semi-simple uniformly closed subalgebra of 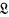(X) that contains some non-zero compact operator, then 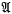has minimal one-sided ideals. The proof depends heavily on the fact (see e.g. Bonsall (3)) that the spectral projection at a non-zero eigenvalue of a compact operator T belongs to the least closed subalgebra of 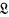(X) that contains T. The uniform norm is quite critical for Bonsall's result, but we are able to give a mild generalization which leads to conditions for the existence of minimal one-sided ideals in subalgebras of 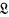(X, Y,〈,〉) where (X, Y,〈,〉) are Banach spaces in normed duality.