Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-11-23T22:33:10.699Z Has data issue: false hasContentIssue false
  • English
  • Français

Vieta’s product for pi from the Archimedean algorithm

Published online by Cambridge University Press:  25 August 2015

Thomas J. Osler  and
Sky Waterpeace
Thomas J. Osler
Affiliation:
Mathematics Department, Rowan University, Glassboro NJ 08028 USA e-mail: [email protected]
Sky Waterpeace
Affiliation:
Mathematics Department, Rowan University, Glassboro NJ 08028 USA e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Extract

In this paper we show how to derive Vieta’s famous product of nested radicals for π [1] from the Archimedean iterative algorithm for π, [2, 3]. Only simple algebraic manipulations are needed.

The Archimedean iterative algorithm for calculating π uses a method involving the two equations:

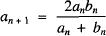
and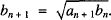

In using this algorithm, we start with a circle of diameter 1. Imagine two regular polygons each with the same number of sides, circumscribed and inscribed to this circle. The larger one has perimeter a0, the smaller has perimeter b0. Since the perimeter of the circle is π we have b0 < π < a0. Now consider regular polygons with twice the number of sides that circumscribe and inscribe the circle and call their perimeters a1 and b1 respectively. These can be calculated from (1) and (2). Continuing in this way we generate the perimeters of inscribed and circumscribed regular polygons, and in each case the number of sides is twice the sides of the previous polygon. Clearly the sequence {an} approaches π from above and {bn} approaches π from below.

Type
Research Article
Information
The Mathematical Gazette , Volume 98 , Issue 543 , November 2014 , pp. 429 - 431
Copyright
Copyright © The Mathematical Association 2014

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Heath, T.L., The works of Archimedes, Cambridge University Press (2004).Google Scholar
2. Berggren, L., Borwein, J., Borwein, P., Pi: A source book (3rd edn.), Springer, New York (2004).Google Scholar
3. Borwein, J., The life of Pi: from Archimedes to ENIAC and beyond, http://www.carma.newcastle.edu.au/jon/pi-2010.pdf.Google Scholar
4. Vieta, F., Variorum de Rebus Mathematicis Reponsorum Liber VII, (1593) in: Opera Mathematica (reprinted), Georg Olms Verlag, Hildesheim, New York (1970) pp. 398–400 and pp. 436-446.Google Scholar
5. Stillwell, I., Mathematics and its history (3rd edn.), Springer, New York (2010).Google Scholar