Hostname: page-component-599cfd5f84-d4snv Total loading time: 0 Render date: 2025-01-07T08:22:39.182Z Has data issue: false hasContentIssue false
  • English
  • Français

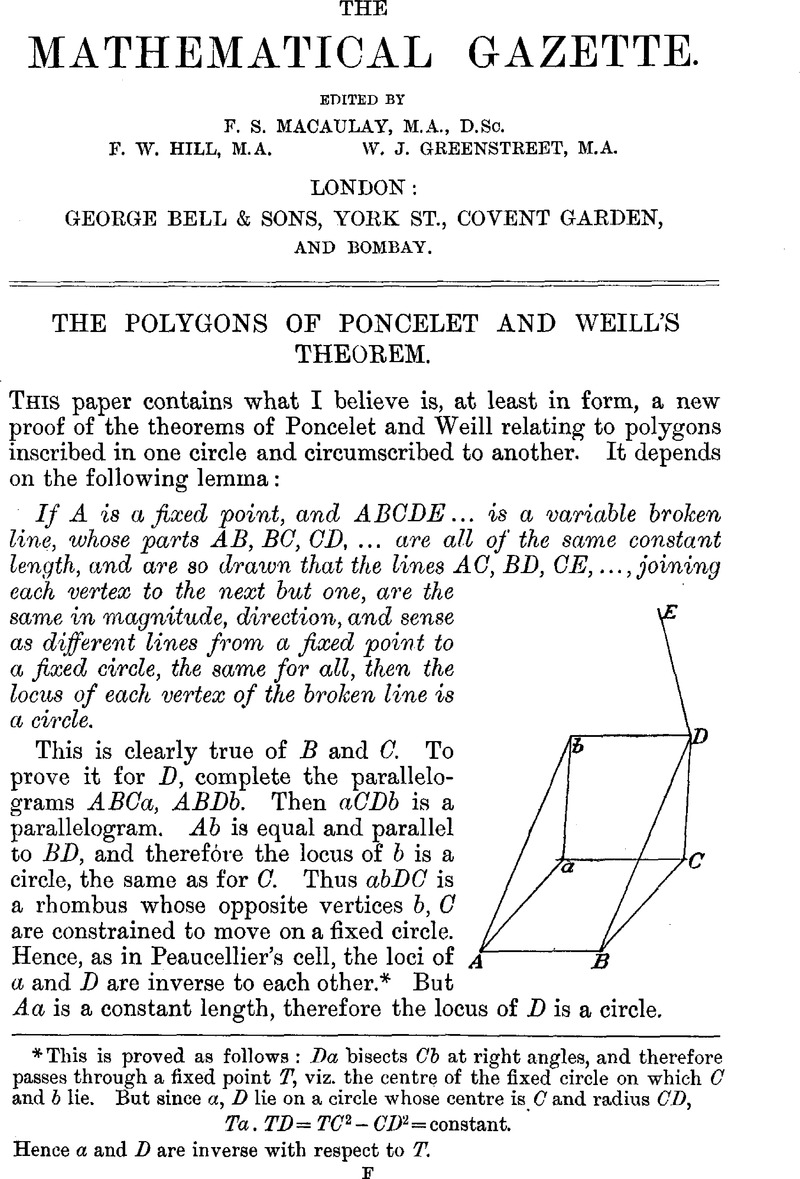
The Polygons of Poncelet and Weill’s Theorem

Published online by Cambridge University Press:  03 November 2016

A. C. Dixon
Get access Rights & Permissions [Opens in a new window]

Abstract

An abstract is not available for this content so a preview has been provided. Please use the Get access link above for information on how to access this content.
Image of the first page of this content. For PDF version, please use the ‘Save PDF’ preceeding this image.'
Type
Other
Information
The Mathematical Gazette , Volume 1 , Issue 12 , October 1897 , pp. 121 - 123
Copyright
Copyright © Mathematical Association 1897

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

page 121 note * This is proved as follows : Da bisects Cb at right angles, and therefore passes through a fixed point T, viz. the centre of the fixed circle on which C and b lie. But since a, D lie on a circle whose centre is C and radius CD,

Ta.TD= TC 2 – CD 2=constant.

Hence a and D are inverse with respect to T.

page 123 note * This is Weill’s Theorem. Compare Casey’s Sequel, p. 164.