Hostname: page-component-745bb68f8f-l4dxg Total loading time: 0 Render date: 2025-01-26T01:40:53.429Z Has data issue: false hasContentIssue false
  • English
  • Français

Groups, conics and recurrence relations

Published online by Cambridge University Press:  03 July 2023

A. F. Beardon
A. F. Beardon*
Affiliation:
D.P.M.M.S., University of Cambridge, Wilberforce Road, Cambridge CB3 0WB e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Extract

In this paper we explore some of the geometry that lies behind the real linear, second order, constant coefficient, recurrence relation(1)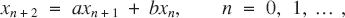where a and b are real numbers. Readers will be familiar with the standard method of solving this relation, and, to avoid trivial cases, we shall assume that ab ≠ 0. The auxiliary equation of t2 = at + b of (1) has two (possibly complex) solutions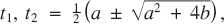and the most general solution of (1) is given by

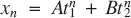  1. (i) when are real and distinct;

  2. (ii) when

  3. (iii)

.

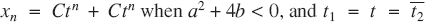
Type
Articles
Information
The Mathematical Gazette , Volume 107 , Issue 569 , July 2023 , pp. 193 - 203
Check for updates
Copyright
© The Authors, 2023. Published by Cambridge University Press on behalf of The Mathematical Association

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Gibson, C. G., Elementary geometry of algebraic curves, Cambridge University Press (1998).Google Scholar
Shirali, S. A., Groups associated with conics, Math. Gaz. 93 (March 2009) pp. 27–41.CrossRefGoogle Scholar
Aharanov, D., Beardon, A. F. and Driver, K. A., Fibonacci, Chebyshev and orthogonal polynomials, Amer. Math. Monthly 112 (2005) pp. 612–630.CrossRefGoogle Scholar
Beardon, A. F., Fibonacci meets Chebyshev, Math. Gaz. 91 (July 2007), pp. 251–255.CrossRefGoogle Scholar
Lewis, B., Trigonometry and Fibonacci numbers, Math. Gaz. 91 (July 2007) pp. 216–226.CrossRefGoogle Scholar
Sporn, H., A group structure on the golden triples, Math. Gaz. 105 (March 2021) pp. 87–97.CrossRefGoogle Scholar
Fukshansky, L., Moore, D., Ohana, R. A. and Zeldow, W., On well-rounded sublattices of the hexagonal lattices, Discrete Mathematics 310 (2010), pp. 3287–3302, and also available at http://arxiv.org/abs/1007.2667CrossRefGoogle Scholar
Dolan, S., The geometric unfolding of recurrence relations, Math. Gaz. 104 (November 2020) pp. 403–411.CrossRefGoogle Scholar