Published online by Cambridge University Press: 03 November 2016
A Little more than three hundred years ago Napier announced to the world his discovery of Logarithms in the Mirifici Logarithmorum Canonis Description “ I have always endeavoured,” we read in one of his works, “ according to my strength and the measure of my ability to do away with the difficulty and tediousness of calculations, the irksomeness of which is wont to deter very many from the study of mathematics. With this aim before me, I undertook the publication of the Canon of Logarithms which I had worked at for a long time in former years; this canon rejected the natural numbers and the more difficult operations performed by them, substituting others which bring out the same results by easy additions, subtractions and divisions by two and by three.” Soon after the publication of the Canon, what he calls “another and better kind of logarithms “occurred to him, practically logarithms to the base 10, as we now know them, and a similar change in his system also suggested itself to Briggs, then Gresham Professor of Geometry in London, one of the first to recognise the immense value of Napier’s discovery In the interval between 1614 and Napier’s death in 1617, they had devised several methods for the construction of the new Tables; but owing to Napier’s failing health he had decided to leave the actual computation to Briggs. As early as 1617 Briggs was able to publish the logarithms of the numbers from 1 to 1000 to eight places. In 1624 he followed this with the numbers from 1 to 20,000 and 90,000 to 100,000 to fourteen places. In 1628 Vlacq, a Dutchman, published the logarithms of all numbers from 1 to 100,000 to ten places, along with a Table of the Logarithms of the Trigonometrical Ratios for every minute, to the same number of places. These Tables of Briggs and Vlacq have never been superseded—their work was done for all time. They contain errors which have gradually been discovered and corrected, but all the Tables that have been published since their time, with one single exception, have been copied, directly or indirectly, from their work.
The publication of this paper has been unfortunately delayed. The author intended it to appear at the time of the Napier Ter-Centenary Celebrations.
page 76 note † (Edinburgh, 1614.) English translation by Wright (London, 1616), and by Filipowski (Edinburgh, 1857).
page 76 note ‡ Quoted in Macdonald’s translation of the Mirifici Logarithmorum Canonis Constructio, p. 88. (Edinburgh, 1889.)
page 76 note § Natural logarithms, Napierean logarithms as they are sometimes called, are not the logarithms known to Napier.
page 77 note * Cf. Glaisher, Phil. Mag. (4), vol. 44, p. 291 (1872).
page 77 note † It is because Briggs and Vlacq used Tables of the Trigonometrical Ratios in which the radius was taken as 1010 that the characteristics 10, 9, 8, etc., appear in the Tables of Logarithmic Sines, Cosines, etc. In common with many other writers of text-books on Trigonometry. I regret to say that I have been guilty of the mistake of saying that 10 is added to the logarithms of the trigonometrical ratios to avoid the inconvenience of printing negative characteristics.
page 77 note ‡ Cf. Macdonald, loc. cit. p. 88.
page 77 note § American Mathematical Monthly, vol. xx. p. 7 (1913). Also Tropfke, Geschichte der Elementar-Mathematik, vol. ii. pp. 148-150 (Leipzig, 1903).
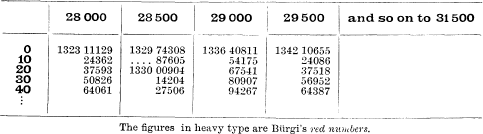
page 78 note * Bürgi’s work was entitled Arithmetische und Geometrische Progress-tabulen, sambt gründ-lichen unterricht, wie solche nützlich in allerley Rechinungen zu gebrauchen und verstanden werden sol (Prague, 1620). But the promised explanation of the Tables is not contained in the volume. A MSS. with this explanation was found at Dantzig about 1850. The numbers of the A.p. he calls the red numbers and prints them in red. The numbers of the G.P., he calls the black numbers, and prints them in that colour. The product of any two black numbers can be obtained by taking the sum of the two corresponding red numbers and then reading off from the Table the black number which corresponds to that sum, multiplying this black number by 10s. For intermediate numbers interpolation would be required, but the series is supposed to have been calculated so far as to make the rule of proportional parts applicable. An extract from Bürgi’s Table is given below:
page 80 note * Napier has 9 995 001.222 927. This mistake is carried forward into the Radical Table of § 11, and some of the Logarithms of his Canon are affected by it.