No CrossRef data available.
Article contents
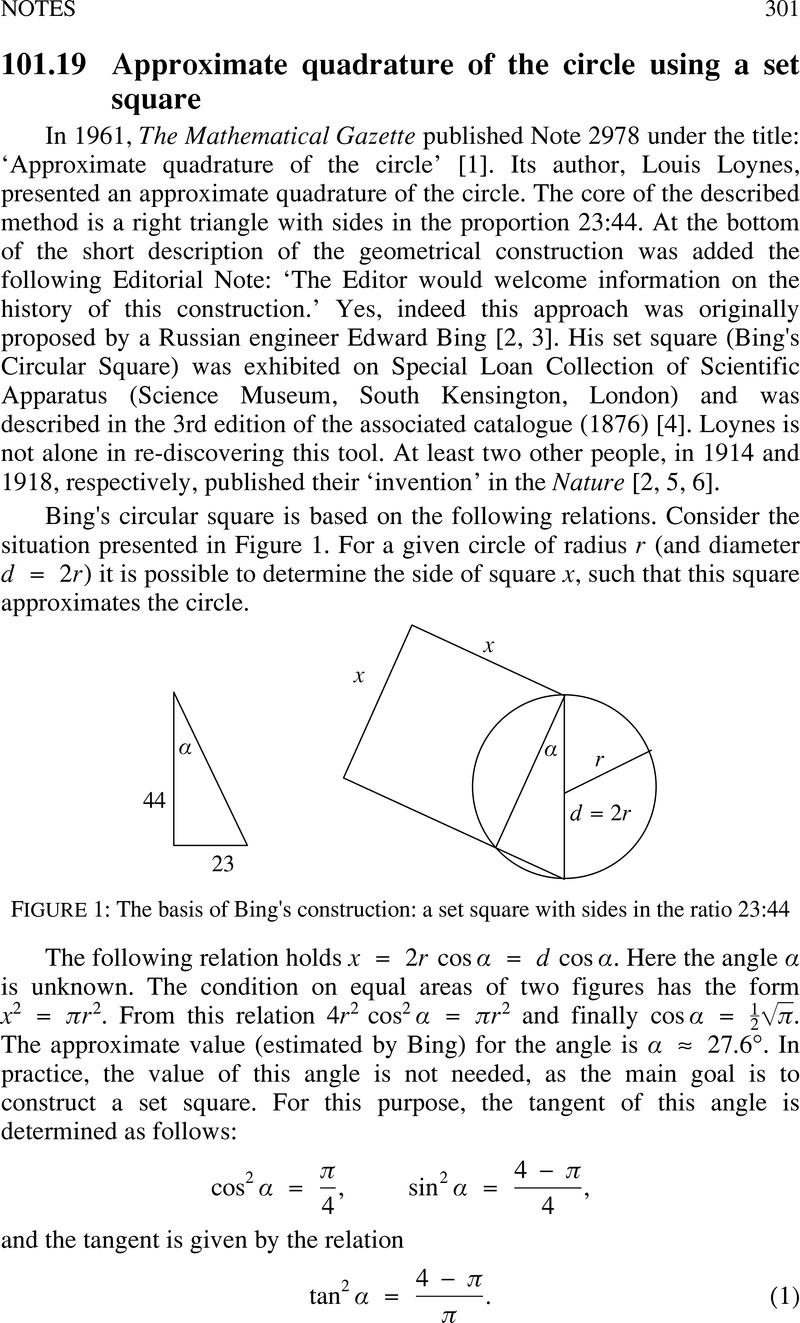
101.19 Approximate quadrature of the circle using a set square
Published online by Cambridge University Press: 15 June 2017
Abstract
An abstract is not available for this content so a preview has been provided. Please use the Get access link above for information on how to access this content.
- Type
- Notes
- Information
- Copyright
- Copyright © Mathematical Association 2017
References
1.
Loynes, Louis, Approximate quadrature of the circle. Math. Gaz.
45 (December 1961) p. 330.Google Scholar
2.
Szyszkowicz, Mieczysław, Krótka historia ekierki Binga (in Polish: A short history of Bing's set square). East European Scientific Journal (EESJ
12 (2) (2016) pp. 57–60 and also available at http://eesj-science.com/wp-content/uploads/2016/09/EESJ_12_2.pdf
Google Scholar
3.
Bing, E., Der Kreiswinkel. Vermischtes. VDI-Z: Zeitschrift für die Entwicklung
, Konstruktion, Produktion, 21 (6) (Juniheft 1877) pp. 273–279.Google Scholar
4.
Smeal, G., Baxandall, D., Construction for an approximate quadrature of the circle, Nature,
101 (2538), (1918) p. 304.CrossRefGoogle Scholar
5.
Hughes, T. M. P., G. B. Mathews, , A triangle that gives the area and circumference of a circle, and the diameter of a circle equal in area to any given square, Nature, 93 (2318), (1914) p. 110.Google Scholar
6.
Baynes, R., Construction for an approximate quadrature of the circle. Nature, 101(2536), (1918) p. 264.CrossRefGoogle Scholar


