Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-26T07:40:13.211Z Has data issue: false hasContentIssue false
  • English
  • Français

Examples of CM curves of genus two defined over the reflex field

Part of: Arithmetic algebraic geometry Computational number theory Abelian varieties and schemes

Published online by Cambridge University Press:  01 August 2015

Florian Bouyer  and
Marco Streng
Florian Bouyer
Affiliation:
University of Warwick, United Kingdom email [email protected]
Marco Streng
Affiliation:
Universiteit Leiden, Netherlands email [email protected]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Van Wamelen [Math. Comp. 68 (1999) no. 225, 307–320] lists 19 curves of genus two over $\mathbf{Q}$ with complex multiplication (CM). However, for each curve, the CM-field turns out to be cyclic Galois over 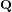$\mathbf{Q}$, and the generic case of a non-Galois quartic CM-field did not feature in this list. The reason is that the field of definition in that case always contains the real quadratic subfield of the reflex field.

We extend Van Wamelen’s list to include curves of genus two defined over this real quadratic field. Our list therefore contains the smallest ‘generic’ examples of CM curves of genus two.

We explain our methods for obtaining this list, including a new height-reduction algorithm for arbitrary hyperelliptic curves over totally real number fields. Unlike Van Wamelen, we also give a proof of our list, which is made possible by our implementation of denominator bounds of Lauter and Viray for Igusa class polynomials.

MSC classification

Primary: 11G15: Complex multiplication and moduli of abelian varieties 14K22: Complex multiplication
Secondary: 11Y99: None of the above, but in this section 11G05: Elliptic curves over global fields 11G07: Elliptic curves over local fields
Type
Research Article
Information
LMS Journal of Computation and Mathematics , Volume 18 , Issue 1 , 2015 , pp. 507 - 538
Copyright
© The Author(s) 2015 

References

Bisson, G. and Streng, M., ‘On polarised class groups of orders in quartic CM-fields’, Preprint, 2013, arXiv:1302.3756.Google Scholar
Bosma, W., Cannon, J. and Playoust, C., ‘The Magma algebra system I: The user language’, J. Symbolic Comput. 24 (1997) no. 3–4, 235–265; Computational algebra and number theory (London, 1993).CrossRefGoogle Scholar
Bruinier, J. H. and Yang, T., ‘CM-values of Hilbert modular functions’, Invent. Math. 163 (2006) no. 2, 229–288.CrossRefGoogle Scholar
Cardona, G. and Quer, J., ‘Field of moduli and field of definition for curves of genus 2’, Computational aspects of algebraic curves , Lecture Notes in Computer Science 13 (World Scientific, 2005) 71–83.CrossRefGoogle Scholar
Carls, R., Kohel, D. and Lubicz, D., ‘Higher-dimensional 3-adic CM construction’, J. Algebra 319 (2008) no. 3, 971–1006.CrossRefGoogle Scholar
Cassels, J. W. S. and Flynn, E. V., Prolegomena to a middlebrow arithmetic of curves of genus 2, Vol. 230 (Cambridge University Press, 1996).CrossRefGoogle Scholar
Cox, D. A., Primes of the form x 2 + ny 2 (John Wiley & Sons, 1989).Google Scholar
Eisenträger, K. and Lauter, K., ‘A CRT algorithm for constructing genus 2 curves over finite fields’, Arithmetics, geometry, and coding theory (AGCT 2005) , Séminaires et Congrès 21 (Société Mathématique de France, Paris, 2010) 161–176; arXiv:math/0405305.Google Scholar
Frey, G. and Lange, T., ‘Complex multiplication’, Handbook of elliptic and hyperelliptic curve cryptography (eds Cohen, H., Frey, G., Avanzi, R., Doche, C., Lange, T., Nguyen, K. and Vercauteren, F.; Chapman & Hall/CRC, 2006) 455–473.Google Scholar
Gaudry, P., Houtmann, T., Kohel, D., Ritzenthaler, C. and Weng, A., ‘The 2-adic CM method for genus 2 curves with application to cryptography’, Advances in cryptology – ASIACRYPT 2006 , Lecture Notes in Computer Science 4284 (Springer, 2006) 114–129.CrossRefGoogle Scholar
Gaudry, P., Kruppa, A., Morain, F., Muller, L., Thome, E. and Zimmermann, P., CADO-NFS 1.1, An implementation of the number field sieve method, http://cado-nfs.gforge.inria.fr/.Google Scholar
Goren, E. Z. and Lauter, K., ‘Class invariants for quartic CM fields’, Ann. Inst. Fourier (Grenoble) 57 (2007) no. 2, 457–480.CrossRefGoogle Scholar
Goren, E. Z. and Lauter, K., ‘Genus 2 curves with complex multiplication’, Int. Math. Res. Not. IMRN 2012 (2012) no. 5, 1068–1142.CrossRefGoogle Scholar
Hindry, M. and Silverman, J. H., Diophantine geometry , Graduate Texts in Mathematics 201 (Springer, New York, 2000).CrossRefGoogle Scholar
Hitt, L., McGuire, G., Naehrig, M. and Streng, M., ‘A CM construction for curves of genus 2 with p-rank 1’, J. Number Theory 131 (2011) no. 5, 920–935; arXiv:0811.3434.CrossRefGoogle Scholar
Igusa, J.-I., ‘Arithmetic variety of moduli for genus two’, Ann. of Math. (2) 72 (1960) no. 3, 612–649.CrossRefGoogle Scholar
Kohel, D. et al. , ‘ECHIDNA algorithms for algebra and geometry experimentation,’ 2007,http://echidna.maths.usyd.edu.au/∼kohel/dbs/complex_multiplication2.html.Google Scholar
Lang, S., Complex multiplication , Grundlehren der mathematischen Wissenschaften 255 (Springer, 1983).CrossRefGoogle Scholar
Lauter, K. and Viray, B., ‘An arithmetic intersection formula for denominators of Igusa class polynomials’, Amer. J. Math. 137 (2015) no. 2, 497–533; doi:10.1353/ajm.2015.0010; arXiv:1210.7841v1.CrossRefGoogle Scholar
Liu, Q., ‘Modèles entiers des courbes hyperelliptiques sur un corps de valuation discrète’, Trans. Amer. Math. Soc. 348 (1996) no. 11, 4577–4610.CrossRefGoogle Scholar
Mestre, J.-F., ‘Construction de courbes de genre 2 à partir de leurs modules’, Effective methods in algebraic geometry (Castiglioncello, 1990) , Progress in Mathematics 94 (Birkhäuser, Boston, 1991) 313–334.CrossRefGoogle Scholar
Milne, J. S., ‘Abelian varieties defined over their fields of moduli, I’, Bull. Lond. Math. Soc. 4 (1972) 370–372.CrossRefGoogle Scholar
Murabayashi, N. and Umegaki, A., ‘Determination of all Q -rational CM-points in the moduli space of principally polarized abelian surfaces’, J. Algebra 235 (2001) no. 1, 267–274.CrossRefGoogle Scholar
The PARI Group, Bordeaux, PARI/GP, version 2.4.3, 2011, http://pari.math.u-bordeaux.fr/.Google Scholar
Shimura, G., ‘On the zeta function of an abelian variety with complex multiplication’, Ann. of Math. (2) 94 (1971) no. 2, 504–533.CrossRefGoogle Scholar
Shimura, G., Introduction to the arithmetic theory of automorphic functions (Princeton University Press, 1994).Google Scholar
Shimura, G., Abelian varieties with complex multiplication and modular functions (Princeton University Press, 1998); sections 1–16 essentially appeared before in [ 28 ].CrossRefGoogle Scholar
Shimura, G. and Taniyama, Y., Complex multiplication of abelian varieties and its applications to number theory , Publications of the Mathematical Society of Japan 6 (The Mathematical Society of Japan, Tokyo, 1961).Google Scholar
Stein, W. et al. , Sage mathematics software 4.7.2, 2011, http://www.sagemath.org/.Google Scholar
Stoll, M. and Cremona, J. E., ‘On the reduction theory of binary forms’, J. Reine Angew. Math. 565 (2003) 79–99.Google Scholar
Streng, M., Sage package for using Shimura’s reciprocity law for Siegel modular functions,http://www.math.leidenuniv.nl/∼streng/recip/.Google Scholar
Streng, M., ‘Complex multiplication of abelian surfaces’, PhD Thesis, Universiteit Leiden, 2010,http://hdl.handle.net/1887/15572.Google Scholar
Streng, M., ‘An explicit reciprocity law for Siegel modular functions’, Preprint, 2011, arXiv:1201.0020.Google Scholar
Streng, M., ‘Computing Igusa class polynomials’, Math. Comp. 83 (2014) 275–309; arXiv:0903.4766.CrossRefGoogle Scholar
Streng, M. and Bouyer, F., ‘Implementation of Mestre’s algorithm in Sage’,  http://trac.sagemath.org/sage_trac/ticket/6341.Google Scholar
Streng, M. and Bouyer, F., ‘Sage code for reduction of hyperelliptic curves, and data files for “Examples of CM curves of genus two defined over the reflex field”’, https://bitbucket.org/mstreng/reduce.Google Scholar
van der Geer, G., Hilbert modular surfaces , Ergebnisse der Mathematik und ihrer Grenzgebiete (3) 16 (Springer, 1988).CrossRefGoogle Scholar
van Wamelen, P., ‘Examples of genus two CM curves defined over the rationals’, Math. Comp. 68 (1999) no. 225, 307–320.CrossRefGoogle Scholar
van Wamelen, P., ‘Proving that a genus 2 curve has complex multiplication’, Math. Comp. 68 (1999) no. 228, 1663–1677.CrossRefGoogle Scholar
Yang, T., ‘Arithmetic intersection on a Hilbert modular surface and the Faltings height’, Asian J. Math. 17 (2013) no. 2, 335–381; doi:10.4310/AJM.2013.v17.n2.a4; arXiv:1008:1854.CrossRefGoogle Scholar
Zimmermann, P. et al. , GMP-ECM 6.4.2 (elliptic curve method for integer factorization), 2012,https://gforge.inria.fr/projects/ecm/.Google Scholar