Hostname: page-component-cd9895bd7-hc48f Total loading time: 0 Render date: 2024-12-26T16:00:41.740Z Has data issue: false hasContentIssue false
  • English
  • Français

GV/cm scale laser-magnetic resonant acceleration in vacuum

Published online by Cambridge University Press:  08 August 2017

Y. Zhang ,
J.-L. Jiao ,
B. Zhang ,
Z.-M. Zhang  and
Y.-Q. Gu
Y. Zhang
Affiliation:
Science and Technology on Plasma Physics Laboratory, Laser Fusion Research Center, China Academy of Engineering Physics (CAEP), Mianyang 621900, China
J.-L. Jiao
Affiliation:
Science and Technology on Plasma Physics Laboratory, Laser Fusion Research Center, China Academy of Engineering Physics (CAEP), Mianyang 621900, China
B. Zhang
Affiliation:
Science and Technology on Plasma Physics Laboratory, Laser Fusion Research Center, China Academy of Engineering Physics (CAEP), Mianyang 621900, China
Z.-M. Zhang
Affiliation:
Science and Technology on Plasma Physics Laboratory, Laser Fusion Research Center, China Academy of Engineering Physics (CAEP), Mianyang 621900, China
Y.-Q. Gu*
Affiliation:
Science and Technology on Plasma Physics Laboratory, Laser Fusion Research Center, China Academy of Engineering Physics (CAEP), Mianyang 621900, China IFSA Collaborative Innovation Center, Shanghai Jiao Tong University, Shanghai 200240, China HEDPs, Center for Applied Physics and Technology Peking University, Beijing 100871, China
*
*Address correspondence and reprint requests to: Y.-q. Gu, Science and Technology on Plasma Physics Laboratory, Laser Fusion Research Center, China Academy of Engineering Physics (CAEP), Mianyang 621900, China; IFSA Collaborative Innovation Center, Shanghai Jiao Tong University, Shanghai 200240, China and HEDPs, Center for Applied Physics and Technology Peking University, Beijing 100871, China. E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Resonant acceleration of electrons by a laser in the background of an extra longitudinal magnetic field is investigated analytically and numerically. The resonant condition is independent of laser intensity, and when satisfied, the energy gain is proportional to 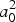$a_0^2 $ and the square of phase difference. This process is mainly limited by the magnitude and spatial size of the extra magnetic field. Under the laboratory conditions, simulation results show that a monoenergetic and collimated electron bunch can still be obtained in ~ GV/cm scale, which sheds a light on the vacuum table-top laser-driven electron accelerators.

Keywords

Electron accelerationExtra magnetic fieldLMRA
Type
Research Article
Information
Laser and Particle Beams , Volume 35 , Issue 3 , September 2017 , pp. 520 - 527
Copyright
Copyright © Cambridge University Press 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Chen, Z., Ho, Y.K., Kong, Q., Wang, P.X., Wang, W. & Xu, J.J. (2007). Two staged laser acceleration with a static magnetic field. J. Appl. Phys. 102, 043110.Google Scholar
Esarey, E., Sprangle, P., Krall, J. & Ting, A. (1996). Overview of plasma-based accelerator concepts. IEEE Trans. Plasma Sci. 24, 252.Google Scholar
Fujioka, S., Zhang, Z., Ishihara, K., Shigemori, K., Hironaka, Y., Johzaki, T., Sunahara, A., Yamamoto, N., Nakashima, H., Watanabe, T., Shiraga, H., Nishimura, H. & Azechi, H. (2013). Kilotesla magnetic field due to a capacitor-coil target driven by high power laser. Sci. Rep. 3, 1170.Google Scholar
Ghotra, H.S. & Kant, N. (2015). Electron acceleration by a chirped laser pulse in vacuum under the influence of magnetic field. Opt. Rev. 22, 539.CrossRefGoogle Scholar
Ghotra, H.S. & Kant, N. (2016 a). Electron injection for direct acceleration to multi-GeV energy by a Gaussian laser field under the influence of axial magnetic field. Phys. Plasma 23, 053115.Google Scholar
Ghotra, H.S. & Kant, N. (2016 b). Multi-GeV electron acceleration by a periodic frequency chirped radially polarized laser pulse in vacuum. Laser Phys. Lett. 13, 065402.CrossRefGoogle Scholar
Ghotra, H.S. & Kant, N. (2016 c). TEM modes influenced electron acceleration by Hermite-Gaussian laser beam in plasma. Laser Part. Beams 34, 385.Google Scholar
Gibbon, P. (2005). Short Pulse Laser Interactions with Matter an Introduction. London: Imperial College Press.CrossRefGoogle Scholar
Gupta, D.N., Kant, N., Kim, D.E. & Suk, H. (2007). Electron acceleration to GeV energy by a radially polarized laser. Phys. Lett. A 368, 402.Google Scholar
Gupta, D.N. & Ryu, C.-M. (2005). Electron acceleration by a circularly polarized laser pulse in the presence of an obliquely incident magnetic field in vacuum. Phys. Plasma 12, 053103.Google Scholar
Harternann, F.V., Fochs, S.N., Sage, G.P.L., Luhmann, N.C. Jr., Woodworth, J.G., Perry, M.D., Chen, Y.J. & Kerman, A.K. (1995). Nonlinear ponderomotive scattering of relativistic electrons by an intense laser field at focus. Phys. Rev. E 51, 4833.Google Scholar
Kawata, S., Maruyama, T., Watanabe, H. & Takahashi, I. (1991). Inverse-bremsstrahlung electron acceleration. Phys. Rev. Lett. 66, 2072.Google Scholar
Leemans, W.P., Gonsalves, A.J., Mao, H.-S., Nakamura, K., Benedetti, C., Schroeder, C.B., Tóth, C., Daniels, J., Mittelberger, D.E., Bulanov, S.S., Vay, J.-L., Geddes, C.G.R. & Esarey, E. (2014). Multi-GeV electron beams from capillary-discharge-guided subpetawatt laser pulses in the self-trapping regime. Phys. Rev. Lett. 113, 245002.Google Scholar
Liu, H., He, X.T. & Chen, S.G. (2004). Resonance acceleration of electrons in combined strong magnetic fields and intense laser fields. Phys. Rev. E 69, 066409.Google Scholar
Lu, W., Tzoufras, M., Joshi, C., Tsung, F.S., Mori, W.B., Vieira, J., Fonseca, R.A. & Silva, L.O. (2007). Generating multi-GeV electron bunches using single stage laser wakefield acceleration in a 3D nonlinear regime. Phys. Rev. Lett. 10, 061301.Google Scholar
Malka, G., Lefebvre, E. & Miquel, J.L. (1997). Experimental observation of electrons accelerated in vacuum to relativistic energies by a high-intensity LaseR. Phys. Rev. Lett. 78, 3314.Google Scholar
Pang, J., Ho, Y.K., Yuan, X.Q., Cao, N., Kong, Q., Wang, P.X. & Shao, L. (2002). Subluminous phase velocity of a focused laser beam and vacuum laser acceleration. Phys. Rev. E 66, 066501.Google Scholar
Pukhov, A., Sheng, Z.M. & Meyer-Ter-vehn, J. (1999). Particle acceleration in relativistic laser channels. Phys. Plasma 6, 2847.Google Scholar
Quesnel, B. & Mora, P. (1998). Theory and simulation of the interaction of ultraintense laser pulses with electrons in vacuum. Phys. Rev. E 58, 3719.Google Scholar
Salamin, Y.I. & Faisal, F.H.M. (1997). Ponderomotive scattering of electrons in intense laser fields. Phys. Rev. A 55, 3678.CrossRefGoogle Scholar
Singh, K.P. (2004). Electron acceleration by a circularly polarized laser pulse in a plasma. Phys. Plasma 11, 3992.CrossRefGoogle Scholar
Singh, K.P. (2006). Acceleration of electrons by a circularly polarized laser pulse in the presence of an intense axial magnetic field in vacuum. J. Appl. Phys. 100, 044907.Google Scholar
Tajima, T. & Dawson, J.M. (1979). Laser electron accelerator. Phys. Rev. Lett. 43, 267.Google Scholar
Wang, P.X., Ho, Y.K., Yuan, X.Q., Kong, Q., Cao, N., Shao, L., Sessler, A.M., Esarey, E., Moshkovich, E., Nishida, Y., Yugami, N., Ito, H., Wang, J.X. & Scheid, S. (2002). Characteristics of laser-driven electron acceleration in vacuum. J. Appl. Phys. 91, 856.Google Scholar
Wong, L.J. & Kärtner, F.X. (2010). Direct acceleration of an electron in infinite vacuum by a pulsed radially-polarized laser beam. Opt. Express 18, 25035.CrossRefGoogle ScholarPubMed
Xiaomingwang, , Zgadzaj, R., Fazel, N., Li, Z., Yi, S.A., Zhang, X., Henderson, W., Chang, Y.-Y., Korzekwa, R., Tsai, H.-E., Pai, C.-H., Quevedo, H., Dyer, G., Gaul, E., Martinez, M., Bernstein, A.C., Borger, T., Spinks, M., Donovan, M., Khudik, V., Shvets, G., Ditmire, T. & Downer, M.C. (2013). Quasi-monoenergetic laser-plasma acceleration of electrons to 2 GeV. Nat. Commun. 4, 2988.Google Scholar