Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-27T07:04:42.307Z Has data issue: false hasContentIssue false
  • English
  • Français

TOWARDS A NON-ARCHIMEDEAN ANALYTIC ANALOG OF THE BASS–QUILLEN CONJECTURE

Part of: Grothendieck groups and $K_0$ Arithmetic problems. Diophantine geometry

Published online by Cambridge University Press:  01 February 2019

Moritz Kerz ,
Shuji Saito  and
Georg Tamme
Moritz Kerz
Affiliation:
Fakultät für Mathematik, Universität Regensburg, 93040Regensburg, Germany ([email protected])
Shuji Saito
Affiliation:
Graduate School of Mathematical Sciences, University of Tokyo, 3-8-1 Komaba, Tokyo, 153-8914, Japan ([email protected])
Georg Tamme
Affiliation:
Fakultät für Mathematik, Universität Regensburg, 93040Regensburg, Germany ([email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

We suggest an analog of the Bass–Quillen conjecture for smooth affinoid algebras over a complete non-archimedean field. We prove this in the rank-1 case, i.e. for the Picard group. For complete discretely valued fields and regular affinoid algebras that admit a regular model (automatic if the residue characteristic is zero) we prove a similar statement for the Grothendieck group of vector bundles 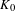$K_{0}$.

Keywords

affinoid algebraPicard grouphomotopy invariance

MSC classification

Primary: 14G22: Rigid analytic geometry
Secondary: 19A49: $K_0$ of other rings
Type
Research Article
Information
Journal of the Institute of Mathematics of Jussieu , Volume 19 , Issue 6 , November 2020 , pp. 1931 - 1946
Check for updates
Copyright
© Cambridge University Press 2019

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The authors are supported by the DFG through CRC 1085 Higher Invariants (Universität Regensburg).

References

Bartenwerfer, W., Die erste ‘metrische’ Kohomologiegruppe glatter affinoider Räume, Nederl. Akad. Wetensch. Proc. Ser. A 40(1) (1978), 1–14.Google Scholar
Bartenwerfer, W., Die höheren metrischen Kohomologiegruppen affinoider Räume, Math. Ann. 241(1) (1979), 11–34.10.1007/BF01406705Google Scholar
Bartenwerfer, W., Holomorphe Vektorraumbündel auf offenen Polyzylindern, J. Reine Angew. Math. 326 (1981), 214–220.Google Scholar
Bartenwerfer, W., Die strengen metrischen Kohomologiegruppen des Einheitspolyzylinders verschwinden, Nederl. Akad. Wetensch. Indag. Math. 44(1) (1982), 101–106.10.1016/1385-7258(82)90011-7Google Scholar
Bosch, S., Lectures on Formal and Rigid Geometry, Lecture Notes in Mathematics, Volume 2105 (Springer, Cham, 2014).Google Scholar
Bourbaki, N., Topological Vector Spaces, Elements of Mathematics (Springer, Berlin, 1987).10.1007/978-3-642-61715-7Google Scholar
Bourbaki, N., Commutative Algebra, Elements of Mathematics (Springer, Berlin, 1989).Google Scholar
Gerritzen, L., Zerlegungen der Picard-Gruppe nichtarchimedischer holomorpher Räume, Compositio Math. 35(1) (1977), 23–38.Google Scholar
Gruson, L., Fibrés vectoriels sur un polydisque ultramétrique, Ann. Sci. Éc. Norm. Supér. 1(4) (1968), 45–89.10.24033/asens.1160Google Scholar
Illusie, L., Laszlo, Y. and Orgogozo, F. (Eds.) Travaux de Gabber sur l’uniformisation locale et la cohomologie étale des schémas quasi-excellents Séminaire à l’Ecole Polytechnique 2006–2008, Astérisque No. 363–364, (SMF, Paris, 2014).Google Scholar
de Jong, J. and van der Put, M., Etale cohomology of rigid analytic spaces, Doc. Math. 1(01) (1996), 1–56.Google Scholar
Kerz, M., Strunk, F. and Tamme, G., Algebraic K-theory and descent for blow-ups, Invent. Math. 211(2) (2018), 523–577.10.1007/s00222-017-0752-2Google Scholar
Kiehl, R., Die de Rham Kohomologie algebraischer Mannigfaltigkeiten über einem bewerteten Körper, Publ. Math. Inst. Hautes Études Sci. 33 (1967), 5–20.Google Scholar
Lindel, H., On the Bass–Quillen conjecture concerning projective modules over polynomial rings, Invent. Math. 65(2) (1981/82), 319–323.10.1007/BF01389017Google Scholar
Lütkebohmert, W., Vektorraumbündel über nichtarchimedischen holomorphen Räumen, Math. Z. 152(2) (1977), 127–143.10.1007/BF01214185Google Scholar
Morrow, M., Pro cdh-descent for cyclic homology and K-theory, J. Inst. Math. Jussieu 15(3) (2016), 539–567.Google Scholar
van der Put, M., Cohomology on affinoid spaces, Compositio Math. 45(2) (1982), 165–198.Google Scholar
van der Put, M. and Schneider, P., Points and topologies in rigid geometry, Math. Ann. 302(1) (1995), 81–103.10.1007/BF01444488Google Scholar
Schneider, P., Points of rigid analytic varieties, J. Reine Angew. Math. 434 (1993), 127–157.Google Scholar
Temkin, M., Desingularization of quasi-excellent schemes in characteristic zero, Adv. Math. 219(2) (2008), 488–522.Google Scholar
Weibel, C., The K-Book, Graduate Studies in Mathematics, Volume 145 (AMS, Providence, RI, 2013).Google Scholar