Article contents
Sur la conjecture de Manin pour certaines surfaces de Châtelet
Published online by Cambridge University Press: 08 March 2013
Résumé
Nous démontrons, sous la forme forte conjecturée par Peyre, la conjecture de Manin pour les surfaces de Châtelet dont les équations sont du type 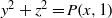 ${y}^{2} + {z}^{2} = P(x, 1)$, où
${y}^{2} + {z}^{2} = P(x, 1)$, où 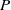 $P$ est une forme binaire quartique à coefficients entiers irréductible sur
$P$ est une forme binaire quartique à coefficients entiers irréductible sur 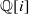 $ \mathbb{Q} [i] $ ou produit de deux formes quadratiques à coefficients entiers irréductibles sur
$ \mathbb{Q} [i] $ ou produit de deux formes quadratiques à coefficients entiers irréductibles sur 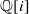 $ \mathbb{Q} [i] $. De plus, nous fournissons une estimation explicite du terme d’erreur de la formule asymptotique sous-jacente. Cela finalise essentiellement la validation de la conjecture de Manin pour l’ensemble des surfaces de Châtelet. La preuve s’appuie sur deux méthodes nouvelles, concernant, du part, les estimations en moyenne d’oscillations locales de caractères sur les diviseurs, et, d’autre part, les majorations de certaines fonctions arithmétiques de formes binaires.
$ \mathbb{Q} [i] $. De plus, nous fournissons une estimation explicite du terme d’erreur de la formule asymptotique sous-jacente. Cela finalise essentiellement la validation de la conjecture de Manin pour l’ensemble des surfaces de Châtelet. La preuve s’appuie sur deux méthodes nouvelles, concernant, du part, les estimations en moyenne d’oscillations locales de caractères sur les diviseurs, et, d’autre part, les majorations de certaines fonctions arithmétiques de formes binaires.
Abstract
We prove Manin’s conjecture, in the strong form conjectured by Peyre, for Châtelet surfaces associated to surfaces of the type  ${y}^{2} + {z}^{2} = P(x, 1)$, where
${y}^{2} + {z}^{2} = P(x, 1)$, where 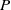 $P$ is a binary quartic form with integer coefficients that is either irreducible over
$P$ is a binary quartic form with integer coefficients that is either irreducible over 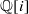 $ \mathbb{Q} [i] $ or the product of two quadratic forms with integer coefficients and irreducible over
$ \mathbb{Q} [i] $ or the product of two quadratic forms with integer coefficients and irreducible over 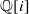 $ \mathbb{Q} [i] $. Moreover, we provide an explicit upper bound for the remainder term in the relevant asymptotic formula. This essentially settles Manin’s conjecture for all Châtelet surfaces. The proof rests on two new tools, namely upper bounds for mean values of local oscillations of characters on divisors and sharp upper estimates for mean values of arithmetic functions of binary forms.
$ \mathbb{Q} [i] $. Moreover, we provide an explicit upper bound for the remainder term in the relevant asymptotic formula. This essentially settles Manin’s conjecture for all Châtelet surfaces. The proof rests on two new tools, namely upper bounds for mean values of local oscillations of characters on divisors and sharp upper estimates for mean values of arithmetic functions of binary forms.
Keywords
MSC classification
- Type
- Research Article
- Information
- Journal of the Institute of Mathematics of Jussieu , Volume 12 , Issue 4 , October 2013 , pp. 759 - 819
- Copyright
- ©Cambridge University Press 2013
References
Bibliographie
- 9
- Cited by


