Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Kaletha, Tasho
2015.
Epipelagic $$L$$ L -packets and rectifying characters.
Inventiones mathematicae,
Vol. 202,
Issue. 1,
p.
1.
Spice, Loren
2018.
Explicit asymptotic expansions for tame supercuspidal characters.
Compositio Mathematica,
Vol. 154,
Issue. 11,
p.
2305.
Adrian, Moshe
and
Kaplan, Eyal
2019.
The Langlands parameter of a simple supercuspidal representation: symplectic groups.
The Ramanujan Journal,
Vol. 50,
Issue. 3,
p.
589.
Lust, Jaime
and
Stevens, Shaun
2020.
On depth zero L‐packets for classical groups.
Proceedings of the London Mathematical Society,
Vol. 121,
Issue. 5,
p.
1083.
Li, Wen-Wei
2020.
Stable conjugacy and epipelagic L-packets for Brylinski–Deligne covers of $${\text {Sp}}(2n)$$.
Selecta Mathematica,
Vol. 26,
Issue. 1,
Feng, Tony
Ronchetti, Niccolò
and
Tsai, Cheng-Chiang
2020.
Epipelagic Langlands parameters and L-packets for unitary groups.
International Journal of Number Theory,
Vol. 16,
Issue. 07,
p.
1449.
Shafiq, Muhammad
Tian, Zhihong
Bashir, Ali Kashif
Jolfaei, Alireza
and
Yu, Xiangzhan
2020.
Data mining and machine learning methods for sustainable smart cities traffic classification: A survey.
Sustainable Cities and Society,
Vol. 60,
Issue. ,
p.
102177.
Adrian, Moshe
and
Kaplan, Eyal
2021.
On The Langlands parameter of a simple supercuspidal representation: even orthogonal groups.
Israel Journal of Mathematics,
Vol. 246,
Issue. 1,
p.
459.
Gross, Benedict H.
2022.
Simple Supercuspidals and the Langlands Correspondence.
Michigan Mathematical Journal,
Vol. 72,
Issue. none,
Oi, Masao
2024.
Simple Supercuspidal 𝐿-Packets of Quasi-Split Classical Groups.
Memoirs of the American Mathematical Society,
Vol. 297,
Issue. 1483,
Romano, Beth
2024.
On input and Langlands parameters for epipelagic representations.
Representation Theory of the American Mathematical Society,
Vol. 28,
Issue. 4,
p.
90.
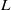 -packets
-packets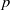 -adic groups, and they conjecture that a special class of these – the simple wild parameters – should correspond to
-adic groups, and they conjecture that a special class of these – the simple wild parameters – should correspond to 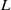 -packets consisting of simple supercuspidal representations. We provide a construction of this correspondence, and show that the simple wild
-packets consisting of simple supercuspidal representations. We provide a construction of this correspondence, and show that the simple wild 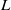 -packets satisfy many expected properties. In particular, they admit a description in terms of the Langlands dual group, and contain a unique generic element for a fixed Whittaker datum. Moreover, we prove their stability on an open subset of the regular semi-simple elements, and show that they satisfy a natural compatibility with respect to unramified base-change.
-packets satisfy many expected properties. In particular, they admit a description in terms of the Langlands dual group, and contain a unique generic element for a fixed Whittaker datum. Moreover, we prove their stability on an open subset of the regular semi-simple elements, and show that they satisfy a natural compatibility with respect to unramified base-change.