Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-27T06:35:24.141Z Has data issue: false hasContentIssue false
  • English
  • Français

RANK TWO TOPOLOGICAL AND INFINITESIMAL EMBEDDED JUMP LOCI OF QUASI-PROJECTIVE MANIFOLDS

Part of: (Co)homology theory Representation theory of groups Homology and cohomology theories Homotopy theory

Published online by Cambridge University Press:  15 February 2018

Stefan Papadima  and
Alexander I. Suciu Open the ORCID record for Alexander I. Suciu [Opens in a new window]
Stefan Papadima
Affiliation:
Simion Stoilow Institute of Mathematics, P.O. Box 1-764, RO-014700 Bucharest, Romania ([email protected])
Alexander I. Suciu
Affiliation:
Department of Mathematics, Northeastern University, Boston, MA 02115, USA ([email protected]) URL: web.northeastern.edu/suciu/
Get access Rights & Permissions [Opens in a new window]

Abstract

We study the germs at the origin of $G$-representation varieties and the degree 1 cohomology jump loci of fundamental groups of quasi-projective manifolds. Using the Morgan–Dupont model associated to a convenient compactification of such a manifold, we relate these germs to those of their infinitesimal counterparts, defined in terms of flat connections on those models. When the linear algebraic group 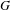$G$ is either 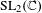$\text{SL}_{2}(\mathbb{C})$ or its standard Borel subgroup and the depth of the jump locus is 1, this dictionary works perfectly, allowing us to describe in this way explicit irreducible decompositions for the germs of these embedded jump loci. On the other hand, if either 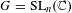$G=\text{SL}_{n}(\mathbb{C})$ for some $n\geqslant 3$, or the depth is greater than 1, then certain natural inclusions of germs are strict.

Keywords

representation varietyvariety of flat connectionscohomology jump locianalytic germdifferential graded algebra modelquasi-projective manifoldadmissible mapDeligne weight filtrationholonomy Lie algebrasemisimple Lie algebra

MSC classification

Primary: 14F35: Homotopy theory; fundamental groups 55N25: Homology with local coefficients, equivariant cohomology
Secondary: 20C15: Ordinary representations and characters 55P62: Rational homotopy theory
Type
Research Article
Information
Journal of the Institute of Mathematics of Jussieu , Volume 19 , Issue 2 , March 2020 , pp. 451 - 485
Copyright
© Cambridge University Press 2018

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The first author’s work was partially supported by the Romanian Ministry of Research and Innovation, CNCS–UEFISCDI, grant PN-III-P4-ID-PCE-2016-0030, within PNCDI III. The second author was partially supported by the Simons Foundation collaboration grant for mathematicians 354156.

†

Deceased 10 January 2018.

References

Arapura, D., Geometry of cohomology support loci for local systems I, J. Algebraic Geom. 6(3) (1997), 563–597; MR 1487227.Google Scholar
Berceanu, B., Măcinic, A., Papadima, S. and Popescu, R., On the geometry and topology of partial configuration spaces of Riemann surfaces, Algebr. Geom. Topol. 17(2) (2017), 1163–1188; MR 3623686.Google Scholar
Budur, N. and Wang, B., Cohomology jump loci of differential graded Lie algebras, Compos. Math. 151(8) (2015), 1499–1528; MR 3383165.Google Scholar
Campana, F., Claudon, B. and Eyssidieux, P., Représentations linéaires des groupes kählériens et de leurs analogues projectifs, J. Éc. Polytech. Math. 1 (2014), 331–342; MR 3322791.Google Scholar
Campana, F., Claudon, B. and Eyssidieux, P., Représentations linéaires des groupes kählériens: factorisations et conjecture de Shafarevich linéaire, Compos. Math. 151(2) (2015), 351–376; MR 3314830.Google Scholar
Corlette, K. and Simpson, C., On the classification of rank-two representations of quasiprojective fundamental groups, Compos. Math. 144(5) (2008), 1271–1331; MR 2457528.Google Scholar
Deligne, P., Griffiths, P., Morgan, J. W. and Sullivan, D., Real homotopy theory of Kähler manifolds, Invent. Math. 29(3) (1975), 245–274; MR 0382702.Google Scholar
Dimca, A. and Papadima, S., Non-abelian cohomology jump loci from an analytic viewpoint, Commun. Contemp. Math. 16(4) (2014), 1350025, 47 pp, MR 3231055.Google Scholar
Dimca, A., Papadima, S. and Suciu, A. I., Alexander polynomials: essential variables and multiplicities, Int. Math. Res. Not. IMRN 2008 (2008), Art. ID rnm119, 36 pp;MR 2416998.Google Scholar
Dimca, A., Papadima, S. and Suciu, A. I., Topology and geometry of cohomology jump loci, Duke Math. J. 148(3) (2009), 405–457; MR 2527322.Google Scholar
Dupont, C., The Orlik–Solomon model for hypersurface arrangements, Ann. Inst. Fourier (Grenoble) 65(6) (2015), 2507–2545; MR 3449588.Google Scholar
Falk, M., Arrangements and cohomology, Ann. Combin. 1(2) (1997), 135–157; MR 1629681.Google Scholar
Goldman, W. and Millson, J., The deformation theory of representations of fundamental groups of compact Kähler manifolds, Publ. Math. Inst. Hautes Études Sci. 67 (1988), 43–96; MR 0972343.Google Scholar
Humphreys, J. E., Introduction to Lie Algebras and Representation Theory, Graduate Texts in Mathematics, Volume 9 (Springer, New York, 1972); MR 0323842.Google Scholar
Humphreys, J. E., Linear Algebraic Groups, Graduate Texts in Mathematics, Volume 21 (Springer, New York-Heidelberg, 1975); MR 0396773.Google Scholar
Kapovich, M. and Millson, J., On representation varieties of Artin groups, projective arrangements and the fundamental groups of smooth complex algebraic varieties, Publ. Math. Inst. Hautes Études Sci. 88 (1998), 5–95; MR 1733326.Google Scholar
Libgober, A. and Yuzvinsky, S., Cohomology of the Orlik–Solomon algebras and local systems, Compos. Math. 121(3) (2000), 337–361; MR 1761630.Google Scholar
Loray, F., Pereira, J. V. and Touzet, F., Representations of quasiprojective groups, flat connections and transversely projective foliations, J. Éc. Polytech. Math. 3 (2016), 263–308; MR 3522824.Google Scholar
Măcinic, A., Papadima, S., Popescu, R. and Suciu, A. I., Flat connections and resonance varieties: from rank one to higher ranks, Trans. Amer. Math. Soc. 369(2) (2017), 1309–1343; MR 3572275.Google Scholar
Morgan, J. W., The algebraic topology of smooth algebraic varieties, Publ. Math. Inst. Hautes Études Sci. 48 (1978), 137–204; MR 0516917.Google Scholar
Papadima, S. and Suciu, A. I., The Milnor fibration of a hyperplane arrangement: from modular resonance to algebraic monodromy, Proc. Lond. Math. Soc. 114(6) (2017), 961–1004; MR 3661343.Google Scholar
Papadima, S. and Suciu, A. I., The topology of compact Lie group actions through the lens of finite models, Int. Math. Res. Notices IMRN. Published electronically athttp://doi.org/10.1093/imrn/rnx294 (2018).Google Scholar
Papadima, S. and Suciu, A. I., Naturality properties and comparison results for topological and infinitesimal embedded jump loci, Preprint, 2016, arXiv:1609.02768v2.Google Scholar
Sullivan, D., Infinitesimal computations in topology, Publ. Math. Inst. Hautes Études Sci. 47 (1977), 269–331; MR 0646078.Google Scholar
Tougeron, J.-Cl., Idéaux de fonctions différentiables, Ergebnisse der Mathematik und ihrer Grenzgebiete, Volume 71 (Springer, Berlin-New York, 1972); MR 0440598.Google Scholar
Whitehead, G. W., Elements of Homotopy Theory, Graduate Texts in Mathematics, Volume 61, (Springer, New York-Berlin, 1978); MR 0516508.Google Scholar
Zuo, K., Representations of Fundamental Groups of Algebraic Varieties, Lecture Notes in Mathematics, Volume 1708 (Springer, Berlin, 1999); MR 1738433.Google Scholar