Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-11-23T22:16:27.572Z Has data issue: false hasContentIssue false
  • English
  • Français

THE NON-COMMUTATIVE KHINTCHINE INEQUALITIES FOR 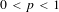$0<p<1$

Part of: Selfadjoint operator algebras Linear spaces and algebras of operators

Published online by Cambridge University Press:  14 October 2015

Gilles Pisier  and
Éric Ricard
Gilles Pisier
Affiliation:
Department of Mathematics, Texas A&M University, College Station, TX 77843-3368, USA ([email protected])
Éric Ricard
Affiliation:
Laboratoire de Mathématiques Nicolas Oresme, Université de Caen Normandie, BP 5186, F 14032, Caen, France ([email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

We give a proof of the Khintchine inequalities in non-commutative 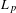$L_{p}$-spaces for all $0<p<1$. These new inequalities are valid for the Rademacher functions or Gaussian random variables, but also for more general sequences, for example, for the analogues of such random variables in free probability. We also prove a factorization for operators from a Hilbert space to a non-commutative $L_{p}$-space, which is new for $0<p<1$. We end by showing that Mazur maps are Hölder on semifinite von Neumann algebras.

Keywords

Non-commutative Lp -spacesKhintchine inequalities

MSC classification

Primary: 46L51: Noncommutative measure and integration 46L07: Operator spaces and completely bounded maps 47L25: Operator spaces (= matricially normed spaces) 47L20: Operator ideals
Type
Research Article
Information
Journal of the Institute of Mathematics of Jussieu , Volume 16 , Issue 5 , November 2017 , pp. 1103 - 1123
Copyright
© Cambridge University Press 2015 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Arazy, J. and Friedman, Y., Contractive projections in C p , Mem. Amer. Math. Soc. 95(459) (1992), vi+109 pp.Google Scholar
Arazy, J. and Lin, P.-K., On p-convexity and q-concavity of unitary matrix spaces, Integral Equations Operator Theory 8 (1995), 295–313.CrossRefGoogle Scholar
Ball, K., Carlen, E. and Lieb, E. H., Sharp uniform convexity and smoothness inequalities for trace norms, Invent. Math. 115 (1994), 463–482.CrossRefGoogle Scholar
Bhatia, R., Matrix Analysis (Springer, New York, 1997).Google Scholar
Buchholz, A., Optimal constants in Khintchine type inequalities for fermions, Rademachers and q-Gaussian operators, Bull. Pol. Acad. 53(3) (2005), 315–321.Google Scholar
Bergh, J. and Löfström, J., Interpolation Spaces. An Introduction (Springer, New York, 1976).Google Scholar
Cobos, F., Peetre, J. and Persson, L. E., On the connection between real and complex interpolation of quasi-Banach spaces, Bull. Sci. Math. 122 (1998), 17–37.CrossRefGoogle Scholar
Davis, W. J., Garling, D. J. H. and Tomczak-Jaegermann, N., The complex convexity of quasinormed linear spaces, J. Funct. Anal. 55 (1984), 110–150.Google Scholar
Dirksen, S. and Ricard, É., Some remarks on noncommutative Khintchine inequalities, Bull. Lond. Math. Soc. 45(3) (2013), 618–624.Google Scholar
Haagerup, U. and Musat, M., On the best constants in noncommutative Khintchine-type inequalities, J. Funct. Anal. 250 (2007), 588–624.CrossRefGoogle Scholar
Haagerup, U. and Pisier, G., Factorization of analytic functions with values in non-commutative L 1 -spaces, Canad. J. Math. 41 (1989), 882–906.Google Scholar
Harcharras, A., Fourier analysis, Schur multipliers on S p and non-commutative 𝛬(p)-sets, Studia Math. 137(3) (1999), 203–260.CrossRefGoogle Scholar
Hiai, F. and Kosaki, H., Means for matrices and comparison of their norms, Indiana Univ. Math. J. 48(3) (1999), 899–936.Google Scholar
Hiai, F. and Kosaki, H., Means of Hilbert Space Operators, Lecture Notes in Mathematics, Volume 1820 (Springer, Berlin, 2003).CrossRefGoogle Scholar
Junge, M. and Parcet, J., Rosenthal’s theorem for subspaces of noncommutative L p , Duke Math. J. 141(1) (2008), 75–122.CrossRefGoogle Scholar
Kosaki, H., Applications of uniform convexity of noncommutative L p -spaces, Trans. Amer. Math. Soc. 283(1) (1984), 265–282.Google Scholar
Kwapień, S., Sums of Independent Banach Space Valued Random Variables (after J. Hoffmann-Jörgensen), Séminaire Maurey–Schwartz 1972–1973: Espaces L p et applications radonifiantes, Exp. no. 6, p. 6 (Centre de Math., École Polytech., Paris, 1973).Google Scholar
Li, D. and Queffélec, H., Introduction à l’étude des espaces de Banach, in Analyse et probabilités, Cours Spécialisés, Vol. 12, xxiv+627 pp. (Société Mathématique de France, Paris, 2004).Google Scholar
Lust-Piquard, F., Inégalités de Khintchine dans C p  (1 < p < ∞), C. R. Acad. Sci. Paris Sér. I 303(7) (1986), 289–292.Google Scholar
Lust-Piquard, F., A Grothendieck factorization theorem on 2-convex Schatten spaces, Israel J. Math. 79(2–3) (1992), 331–365.Google Scholar
Lust-Piquard, F. and Pisier, G., Noncommutative Khintchine and Paley inequalities, Ark. Mat. 29(2) (1991), 241–260.Google Scholar
Lust-Piquard, F. and Xu, Q., The little Grothendieck theorem and Khintchine inequalities for symmetric spaces of measurable operators, J. Funct. Anal. 244(2) (2007), 488–503.CrossRefGoogle Scholar
Maurey, B., Une nouvelle démonstration d’un théorème de Grothendieck, Séminaire Maurey–Schwartz Année 1972–1973: Espaces L p et applications radonifiantes, Exp. no. 22, p. 7 (Centre de Math., École Polytech., Paris, 1973).Google Scholar
Maurey, B., Théorèmes de factorisation pour les opérateurs linéaires à valeurs dans les espaces L p , Astérisque, Volume 11, ii+163 pp. (Société Mathématique de France, Paris, 1974).Google Scholar
Nelson, E., Notes on non-commutative integration, J. Funct. Anal. 15 (1974), 103–116.Google Scholar
Pisier, G., Grothendieck’s theorem for noncommutative C ∗ -algebras, with an appendix on Grothendieck’s constants, J. Funct. Anal. 29(3) (1978), 397–415.Google Scholar
Pisier, G., Introduction to Operator Space Theory, London Mathematical Society Lecture Note Series, Volume 294, viii+478 pp. (Cambridge University Press, Cambridge, 2003).Google Scholar
Pisier, G., Remarks on the non-commutative Khintchine inequalities for 0 < p < 2, J. Funct. Anal. 256 (2009), 4128–4161.CrossRefGoogle Scholar
Pisier, G., Grothendieck’s Theorem, past and present, Bull. Amer. Math. Soc. 49 (2012), 237–323.Google Scholar
Pisier, G. and Xu, Q., Non-commutative L p -spaces, in Handbook of the Geometry of Banach Spaces, Vol. 2, pp. 1459–1517 (North-Holland, Amsterdam, 2003).Google Scholar
Raynaud, Y., On ultrapowers of non commutative L p spaces, J. Operator Theory 48(1) (2002), 41–68.Google Scholar
Ricard, E., Hölder estimates for the noncommutative Mazur maps, Arch. Math. (Basel) 104(1) (2015), 37–45.CrossRefGoogle Scholar
Ricard, E. and Xu, Q., Complex interpolation of weighted non-commutative L p -spaces, Houston J. Math. 37 (2011), 1165–1179.Google Scholar
Ricard, E. and Xu, Q., A noncommutative martingale convexity inequality, Ann. Probab. to appear.Google Scholar
Takesaki, M., Theory of Operator Algebras I (Springer, Berlin, 1979).Google Scholar
Takesaki, M., Theory of Operator Algebras II. Encyclopaedia of Mathematical Sciences, Vol. 125, Operator Algebras and Non-commutative Geometry, Volume 6 (Springer, Berlin, 2003).Google Scholar
Terp, M., L p spaces associated with von Neumann algebras, Notes, Københavns Universitets Matematiske Institut (1981).Google Scholar
Tomczak-Jægermann, N., The moduli of smoothness and convexity and the Rademacher averages of trace classes S p (1⩽p < ∞), Studia Math. 50 (1974), 163–182.Google Scholar
Voiculescu, D., Dykema, K. and Nica, A., Free Random Variables, CRM Monograph Series, Volume 1 (American Mathematical Society, Providence, RI, 1992).Google Scholar
Xu, Q., Applications du théorème de factorisation pour des fonctions à valeurs opérateurs, Studia Math. 95 (1990), 273–292.Google Scholar