Hostname: page-component-78c5997874-xbtfd Total loading time: 0 Render date: 2024-11-09T01:36:50.316Z Has data issue: false hasContentIssue false
  • English
  • Français

MINIMAL HYPERSURFACES ASYMPTOTIC TO SIMONS CONES

Part of: Classical differential geometry

Published online by Cambridge University Press:  01 April 2015

Laurent Mazet
Laurent Mazet*
Affiliation:
Université Paris-Est, LAMA (UMR 8050), UPEC, UPEM, CNRS, 61, avenue du Général de Gaulle, F-94010 Créteil cedex, France ([email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

In this paper, we prove that, up to similarity, there are only two minimal hypersurfaces in $\mathbb{R}^{n+2}$ that are asymptotic to a Simons cone, i.e., the minimal cone over the minimal hypersurface 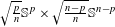$\sqrt{\frac{p}{n}}\mathbb{S}^{p}\times \sqrt{\frac{n-p}{n}}\mathbb{S}^{n-p}$ of 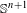$\mathbb{S}^{n+1}$ .

Keywords

minimal hypersurfacesSimons cone

MSC classification

Secondary: 53A10: Minimal surfaces, surfaces with prescribed mean curvature
Type
Research Article
Information
Journal of the Institute of Mathematics of Jussieu , Volume 16 , Issue 1 , February 2017 , pp. 39 - 58
Copyright
© Cambridge University Press 2015 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alencar, H., Barros, A., Palmas, O., Guadalupe Reyes, J. and Santos, W., O (m) × O (n)-invariant minimal hypersurfaces in ℝ m+n , Ann. Global Anal. Geom. 27 (2005), 179–199.CrossRefGoogle Scholar
Alexandrov, A. D., A characteristic property of spheres, Ann. Mat. Pura Appl. (4) 58 (1962), 303–315.CrossRefGoogle Scholar
Allard, W. K. and Almgren, F. J. Jr, On the radial behavior of minimal surfaces and the uniqueness of their tangent cones, Ann. of Math. (2) 113 (1981), 215–265.Google Scholar
Almgren, F. J. Jr, Some interior regularity theorems for minimal surfaces and an extension of Bernstein’s theorem, Ann. of Math. (2) 84 (1966), 277–292.Google Scholar
Bombieri, E., De Giorgi, E. and Giusti, E., Minimal cones and the Bernstein problem, Invent. Math. 7 (1969), 243–268.Google Scholar
Ilmanen, T. and White, B., Sharp lower bounds on density of area-minimizing cones, preprint, 2010, arXiv:1010.5068.Google Scholar
Marques, F. C. and Neves, A., Min-max theory and the Willmore conjecture, Ann. of Math. (2) 179 (2014), 683–782.CrossRefGoogle Scholar
Meeks, W. H. III and Wolf, M., Minimal surfaces with the area growth of two planes: the case of infinite symmetry, J. Amer. Math. Soc. 20 (2007), 441–465.CrossRefGoogle Scholar
Schoen, R. M., Uniqueness, symmetry, and embeddedness of minimal surfaces, J. Differential Geom. 18(1984) (1983), 791–809.Google Scholar
Simon, L., Isolated singularities of extrema of geometric variational problems, in Harmonic Mappings and Minimal Immersions (Montecatini 1984), Lecture Notes in Mathematics, Volume 1161, pp. 206–277 (Springer, Berlin, 1985).CrossRefGoogle Scholar
Simon, L. and Solomon, B., Minimal hypersurfaces asymptotic to quadratic cones in R n+1 , Invent. Math. 86 (1986), 535–551.CrossRefGoogle Scholar
Simons, J., Minimal varieties in riemannian manifolds, Ann. of Math. (2) 88 (1968), 62–105.CrossRefGoogle Scholar
Sogge, C. D., Oscillatory integrals and spherical harmonics, Duke Math. J. 53 (1986), 43–65.CrossRefGoogle Scholar