Article contents
Local quaternionic rigidity for complex hyperbolic lattices
Published online by Cambridge University Press: 01 September 2010
Abstract
Let 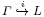 be a lattice in the real simple Lie group L. If L is of rank at least 2 (respectively locally isomorphic to Sp(n, 1)) any unbounded morphism ρ : Γ → G into a simple real Lie group G essentially extends to a Lie morphism ρL : L → G (Margulis's superrigidity theorem, respectively Corlette's theorem). In particular any such morphism is infinitesimally, thus locally, rigid. On the other hand, for L = SU(n, 1) even morphisms of the form
be a lattice in the real simple Lie group L. If L is of rank at least 2 (respectively locally isomorphic to Sp(n, 1)) any unbounded morphism ρ : Γ → G into a simple real Lie group G essentially extends to a Lie morphism ρL : L → G (Margulis's superrigidity theorem, respectively Corlette's theorem). In particular any such morphism is infinitesimally, thus locally, rigid. On the other hand, for L = SU(n, 1) even morphisms of the form  are not infinitesimally rigid in general. Almost nothing is known about their local rigidity. In this paper we prove that any cocompact lattice Γ in SU(n, 1) is essentially locally rigid (while in general not infinitesimally rigid) in the quaternionic groups Sp(n, 1), SU(2n, 2) or SO(4n, 4) (for the natural sequence of embeddings SU(n, 1) ⊂ Sp(n, 1) ⊂ SU(2n, 2) ⊂ SO(4n, 4)).
are not infinitesimally rigid in general. Almost nothing is known about their local rigidity. In this paper we prove that any cocompact lattice Γ in SU(n, 1) is essentially locally rigid (while in general not infinitesimally rigid) in the quaternionic groups Sp(n, 1), SU(2n, 2) or SO(4n, 4) (for the natural sequence of embeddings SU(n, 1) ⊂ Sp(n, 1) ⊂ SU(2n, 2) ⊂ SO(4n, 4)).
MSC classification
- Type
- Research Article
- Information
- Journal of the Institute of Mathematics of Jussieu , Volume 11 , Issue 1 , January 2012 , pp. 133 - 159
- Copyright
- Copyright © Cambridge University Press 2010
References
- 6
- Cited by


